
An assemblage tracing the journey of the Mathematics Section of the ICTP – From its early defining moments recounted by Sharon Berry Laurenti serving as its secretary at the time; a biographical note on James Eells, the first head of the Mathematics Section, by his Warwick University colleague David Elworthy; interviews with mathematicians Alberto Verjovsky, Lothar Göttsche, Claudio Arezzo, Stefano Luzzatto, and Emanuel Carneiro, by Bhāvanā editors Rajesh Akkihal and Mahit Warhadpande; and a postscript by C.S. Aravinda.
Sharon Berry Laurenti

Going right back to the very beginning, Eells was thinking about a name/title for the group, categorically dismissing “Department”, and when I suggested “Section”, he immediately adopted it, thus we became the Mathematics Section. What may seem to some a triviality, he also requested that our seminar announcements, etc., be circulated on coloured paper, the signature blue that distinguished maths announcements from those of the Centre’s other groups.1 Our identity had been established.
our seminar announcements circulated on coloured paper – the signature blue – distinguished maths circulars from those of the Centre’s other groups

Jim was in permanent residence for that first year, accompanied by his wonderful wife, Nan (he seemed to derive great pleasure from introducing her to everyone as “my first wife”). Nan, with Alberto’s equally wonderful wife, Janet, created a women’s group to help integrate the accompanying wives of the Centre’s scientists. Bruna Marcuzzi, a housekeeper at the Galileo guest house at the time, also played an active role in the group, which met weekly. Among their activities were, for example, Italian lessons.

Working with Jim and Alberto was very colourful and fulfilling. And interacting with Nan and Janet was a delight. When, in the mid-nineties, Alberto left, the Section largely lost its soul.
M.S. Narasimhan succeeded Jim as Head of Mathematics in late 1992. I had frequently come across his name through Jim (who fondly referred to him as “MS”), and thus understood that he was a prominent figure in the mathematics world. So, while – for most of 1992 – we were waiting for him to take up residence as the new head of the Section, I was a little anxious as to whether I would be able to live up to his expectations.

It was a rare privilege indeed to have worked alongside Jim, Alberto and Narasimhan, whose leadership I missed after retirement.
I retired in 2009 after 27 years of gratifying work at ICTP.
Sharon Berry Laurenti worked as the secretary of the Mathematics Section for nearly two decades. Together with Alessandra Bergamo, the secretary mainly tasked with organizational matters of the maths workshops and conferences at ICTP, the two of them formed a formidable team working in tandem, turning the maths section corridor to a welcoming home to mathematicians from across the world, donning their roles with remarkable efficiency, and forging lasting friendships.
Kenneth David Elworthy
Born on 25 October 1926 in Cleveland, Ohio, the mathematician James Eells moved to Britain from the United States in the late sixties, becoming Professor of Analysis at Warwick University, and had an important, and continuing, effect on the development of mathematics in his adopted country.
His appreciation of the difficulties faced by scientists, and mathematicians in particular, working in Third World countries led to his becoming in 1986 the first director of a new Mathematics Section at the International Centre for Theoretical Physics at Trieste: one of the centre’s main aims is to give Third World scientists the opportunity to keep up to date with rapidly moving areas of science and mathematics.
Eells went to school at Western Reserve Academy until his exuberance led to his expulsion. However, he was admitted to Bowdoin College, a distinguished liberal arts college in Maine, graduating in 1947. After Bowdoin he had a “gap” year teaching mathematics at Robert College (now Bogaziçi University) in Istanbul. He returned to the US to be an instructor in mathematics at Amherst, during which time he met his future wife, Nan.
After two years he decided that his career really was to be in mathematics and enrolled for a PhD at Harvard under the topologist and analyst Hassler Whitney. In 1954 he, with Nan, two young daughters and a PhD, set off on his academic career. This started at the Institute for Advanced Study at Princeton, went on to the University of California at Berkeley, returned to the East Coast for a position at Columbia University, New York; and in 1964, after a year at Churchill College, Cambridge, he took a full professorship at Cornell University.
He returned to Cambridge for 1966-67; two events that year gave a foretaste of the future. In December he visited Ghana, in connection with Churchill College, to give a mathematics course, having persuaded a Cornell colleague, Cliff Earle, to make a follow-up visit early in 1967. Between the two visits they began fruitful joint research, and Jim Eells was invited to run a small symposium in the summer of 1967 in Warwick, with Earle as one of the principal participants.
The innovative mathematics department of the new university of Warwick had already gained an international reputation and in 1969, excited by the freedoms and potential in Warwick, Eells joined the Mathematics Department there, becoming its first Professor of Analysis.
It was an appointment which fitted perfectly with the philosophy of the department at that time, which was to feature research in global, rather than traditional, analysis; and it was already becoming known as a centre for the global approach to dynamical systems theory. It is tempting to describe global analysis as a holistic approach to mathematics. In it the whole geometry or topology of the spaces involved play a role, rather than just the equations describing the behaviour or motion in small areas. Non-linearity, especially that caused by curvature, is a prevalent aspect. A prime example is Eells’s most famous article, “Harmonic Mappings of Riemannian Manifolds”, published in the American Journal of Mathematics in 1964. Written with J.H. Sampson of Johns Hopkins University, it founded the theory of “harmonic maps” and the “non-linear heat flow”.
His appreciation of the difficulties faced by mathematicians working in Third World countries led to his becoming in 1986 the first director of the maths group at ICTP
The latter describes the behaviour of one shape stretched over another. For example, think of an uninflated balloon wrapped around the surface of a sphere, anchor ring or of some other shape, and allowed to settle down, relaxing, but only moving on the surface. Strange-shaped balloons are also allowed, for example water rings or just a piece of balloon with ends fixed. The final position of minimal stretching would depend on the shape of the balloon, the shape of the surface, and the way it was wrapped. For example, you might force the balloon over the sphere before sealing the end, or you might just lie the balloon slightly stretched over part of the surface. The end result is the “harmonic map”.

Infinite dimensional objects such as the space of all paths on a surface often occur in global analysis, and one of Eells’ early works was to show that many such spaces have a “smooth” structure which enables calculus to be applied on them. He also played a leading role in the classification of such infinite dimensional manifolds. Global analysis and probability theory were combined in foundational work in the analysis of Brownian motion on curved spaces, such as the surface of spheres.
One result was that, if you roll a surface along the trajectory of a typical Brownian path on an ink-covered plane, the mark the ink makes on the surface will be the trajectory of a “Brownian particle on the surface”. A difficulty here is that Brownian particles move so irregularly that standard Newtonian calculus cannot be applied, so even what it means to “roll” is unclear. It has to be replaced by Ito calculus, the calculus which is also now in regular use in mathematical finance. From the mid-Seventies, however, the main part of Eells’ work was related to harmonic map theory.
The highly successful, year-long, Warwick symposia he organized, “Global Analysis” in 1971-72 and “Geometry of the Laplace Operator” in 1976-77, to a large extent introduced novel mathematics to Britain, and together with Eells’ many and energetically pursued connections abroad, attracted fine PhD students and research fellows to study with him. After the first of these, in the summer of 1972, he took the symposium to the International Centre for Theoretical Physics at Trieste for the first session held there on mathematics. This was an early move in the development of a Mathematics Division at ICTP, of which he became the first director, from 1986 to 1992, on partial secondment from Warwick. His research activities in Warwick were barely diminished, however, and if anything there were more visitors and visiting scholars, together with another year-long symposium, “Partial Differential Equations in Differential Geometry”, in 1989-90.
Eells’ mathematical influence internationally became even more substantial, both personally, as a mentor, and scientifically, through the spread of his work and that which was built on it. He had at least 38 graduate students, most of whom became academics. However there are many more throughout the world who owe their careers to him.
The highly successful, year-long, Warwick symposia he organized in 1971-72 and in 1976-77, to a large extent introduced novel mathematics to Britain
Many, together with their families, will also especially remember Nan Eells for her friendship and excellent dinner parties, with only mild attempts to control the exuberance of her husband. Jim Eells was a man of irrepressible enthusiasm for mathematics, and for most other things; especially people, irreverent fun, lots of wine, and music. His interest in the latter ranged across most styles and he would delight the younger children of his colleagues with lively performances of scatological songs.
He retired from Warwick in 1992, moving to Cambridge. He continued working on harmonic maps and travelling abroad to discuss mathematics or for conferences. He also helped to set up a regular UK-Japan Winter School in Mathematics. His final monograph, Harmonic Maps between Riemannian Polyhedra, co-authored with the Danish mathematician B. Fuglede, was published in 2001. Jim Eells died in Cambridge on 14 February 2007, leaving behind a formidable mathematical legacy and memories of several fond friendships across the world.
Kenneth David Elworthy is Professor Emeritus of Mathematics at the University of Warwick. Stochastic analysis, stochastic differential equations and geometric analysis are his main areas of interest.
Interview with Alberto Verjovsky
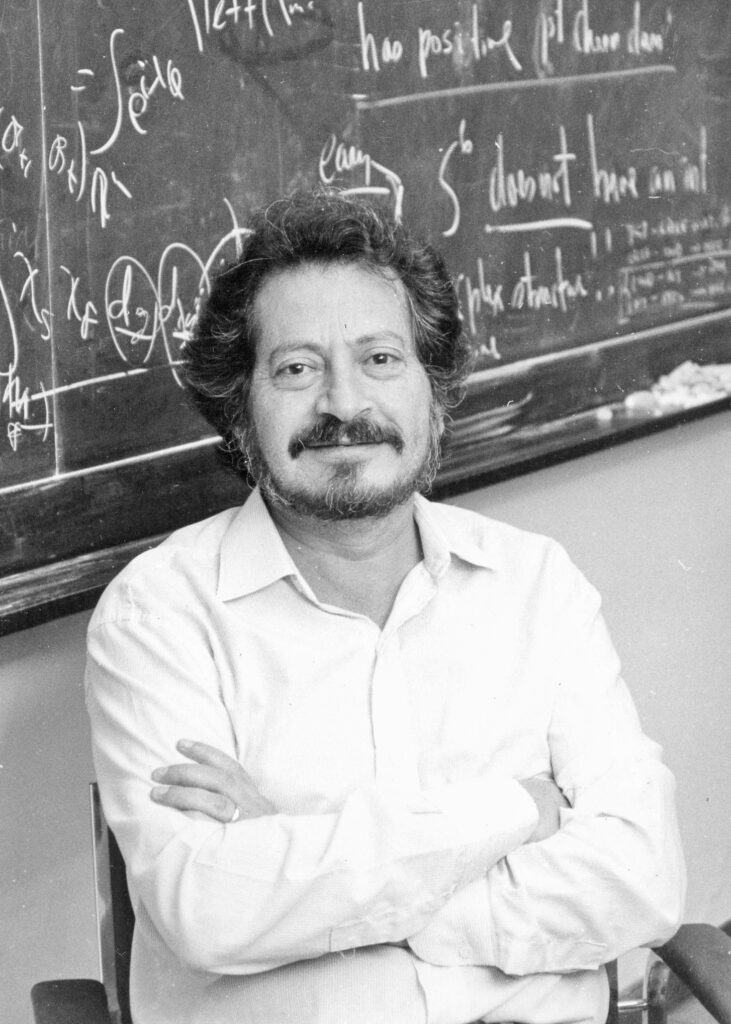
Warm welcome Alberto. Thanks a lot for making time to talk to us. Shall we start by learning a bit about your early life, your schooling, the medium of instruction, and your formative years?
One day, during my first few days in college, I accidentally saw a poster on the campus saying Professor Lefschetz will deliver a seminar on dynamical systems. I attended the seminar. It was a transformative experience. With all modesty, I think I was successful, because one year later I received a letter from him suggesting that I enroll in the programme at Brown University. He lived in Princeton and became professor at Brown. He used to go there weekly, and so I had this opportunity of meeting these great mathematicians. Of course, at that time I wasn’t much aware of it. You know, you are 19 and you see an older man, very wise, but you don’t realize how good they are. Anyhow, after I did my PhD at Brown, though not under his direction but under Mauricio Peixoto, a Brazilian mathematician, who invited me to go to IMPA (Istituto de Matemática Pura e Aplicada) Brazil. At that time, IMPA was playing a role similar to Tata [TIFR] in India. These are two institutions in developing countries which grew to be fantastic places for research. Tata and IMPA are probably some of the very best institutions. And so I was at IMPA and got my PhD. Of course, I returned to Mexico thereafter and started doing my research.

This was before 1986, right?

Indeed yes. We have been experiencing that.

Looks like it was coincidental that Abdus Salam retired in the same year that you left ICTP, in 1993.

Only once I interacted scientifically with him. So I will tell you the story. I was in my office and the secretary, Sharon Laurenti, who is of course retired now and not there anymore, but Laurenti was a super secretary. You know, they say that every good institute has a great secretary. It’s almost an axiom. And so Laurenti informed me, Professor Salam wants to talk to you. And I just thought, wow, maybe the budget of mathematics is going down the drain. So I arrived, knocked at his office, and entered. He said, “I want you to explain to me a little bit of knot theory and braids”. I changed my mindset. I went to the blackboard and started my viewpoint of braids. And said, “well, a braid with two strands only depends on the number of twists. With three strands it’s very complicated. It’s a Braid group. But with two strands it’s like DNA”. And I said that the probability of being left-handed or right-handed, it’s the same thing. Maybe half the DNA is left-handed. “No!”, he said, “all are left-handed”. And he laughed and enjoyed the talk. He gave me an apple. And that was it. About two weeks later, he wrote a paper titled The role of chirality in the origin of life,2 discussing why DNA chooses the left over the right. So that’s my anecdote. I was probably with him for about two hours and that’s my only technical interaction with him. But anyhow, he was a fantastic personality, who had this vision of ICTP.

And then around the time left, came Professor Narasimhan. Another great personality that I met. We used to have coffee. He would explain the stable bundles and all those beautiful concepts and everything to me. I learned so much from him.
I met people from all over the world. I heard many Nobel laureates speak here and I attended fantastic conferences. But the beautiful thing, and the main point, was that it was full of people from developing countries. You could see all races, all religions, everything happening here and it was so peaceful. Because that’s what science is. And that’s a great experience.
I also had a great opportunity to go to India, to Tata,3 only once. Went to Aligarh also. There was a parallel conference. I knew a little bit about André Weil, the great algebraic geometer who went to India and spent some years at the Aligarh University when he was young. I think he spoke Sanskrit. So he loved the culture of India. The one thing I remember seeing there was the library, some books that were obviously chosen by him, I think. All in all, it was a great experience.
Could you tell us a little about your parents and childhood?
And Santiago in your name is…?
My father had just finished high school education. But he read a lot and he became an expert in coins. He was the president of the Numismatic Society of Mexico. The first coins in the American continent were coined in Mexico with silver. Mexico was producing silver. He told me an anecdote that later I verified. In the 16th, 17th, 18th, and 19th, beginning of the 19th century, the equivalent of the dollar was the Mexican peso. And the reason for that is because it was a silver coin whose value mattered. Now the dollar is all artificial. It’s like Bitcoin. But at that time, the coin was important. And it circulated in Asia. When I was in Vietnam in 1990 for a conference in mathematics, maybe the first maths conference after the Vietnam war, I went to the market, a souvenir shop, and I bought some coins from Mexico. It was true what my father had told me.
they say that every good institute has a great secretary
My mother studied until sixth grade. So I became the first doctor, which is very typical of a developing country then. But now, people are more advanced. So I was in a family where my mother divorced and I had a stepfather who loved mathematics. And he had old books, including the book which was used by Ramanujan, the great hero of ours. It’s a British book, by G.S. Carr, titled “A synopsis of elementary results in mathematics”. The book is actually not a good book in mathematics. It has beautiful problems. I have it. But of course, Ramanujan was a genius, so it didn’t matter which book he read. This book that I got from my father was not in good condition, but I learned a little bit, that’s what motivated my studying mathematics. I remember that there was a chapter, on permutations and combinatorics, how many ways can five people fit in an auditorium of 50. I was fascinated by that. I didn’t know there was a profession of mathematician. So, you went to engineering, and that’s what I did too. And one day a friend comes and says, hey, here next door, there is the faculty of science. And it was fantastic, you know, they really taught you what an integral is. Great teachers, and they had great coffee there. So, I decided to study mathematics. And at the beginning, I studied both engineering and mathematics, and then I abandoned engineering and dedicated myself to mathematics. But I like to say as a joke, which is true, is when I was in engineering, I was very good at integration. Of course, cosines, sines, and I used to change variables, but I didn’t know what an integral was. Then I went to mathematics, and I knew what an integral was, but I never integrated (laughs). So, that’s more or less my trajectory, my family, my middle class background, and that at least one member got a PhD, which I’m very proud of.
Could you please tell us about your PhD thesis?

You mainly continued to work in dynamical systems, it appears.

Well, I thought I proved that the Riemann hypothesis was false. Then I came to my house and I telephoned Dennis Sullivan who was in New York. “Hey, Dennis, I think I proved that the Riemann hypothesis is false”. The answer at the other end is silence. “Is Janet around?” he asked. Janet is My wife (laughs). And I tried to respond in the most intelligent way, saying that Janet went to work and blah, blah. Then I explained that I was serious. It was actually based on the work by Dani on horocycle flows. Dennis then told me, “okay, I pay you the ticket to come to New York to visit me”. That was great because at that time with my meagre salary, I wouldn’t have been able to go to New York. Maybe the price of the ticket was three times my salary. Anyway, I went and learnt that I wasn’t completely wrong. But the thing was that there was a problem of regularity, related to lattice point counting. And so, there ended my adventure with the Riemann hypothesis.
I never heard anyone who doesn’t feel gratitude for going to ICTP
Anyhow, I published two papers related to that. A small contribution, but it is just to tell you that one can go from dynamical systems to number theory, to algebraic geometry, and holomorphic foliations. My approach has been trying to learn about many things. When I teach in my class, that’s one of my primary messages. Everything is interconnected with everything. And if they invite you to a conference and you don’t understand the title, and you say, I don’t go. You are wrong. Sometimes you go to a conference, totally disconnected apparently. Then the speaker gives you an idea for your work. Now, of course, with the computers and the facility of information, we don’t go to the library anymore. At least, not as much as we did. You go to the library looking for a certain book, but it so happens that next to this book there’s another book which calls your attention, you take the other one, you open it, it turns out that’s the one you needed! The experience of actually smelling the paper and touching it. So, that’s more or less my adventure with mathematics. The only thing I can say about that is that I love my subject.
Just as your experience at ICTP was helpful to you, you have helped many people to come over here…. Could we hear your retrospective of that journey?

As you walk in the corridors of IMPA, you smell mathematics
Could you tell us about any dream problem or a question that you still think about?
I want to thank ICTP for everything it has done for the world
ICTP is celebrating its 60th anniversary. Would you like to say anything about this milestone?

You were conferred the Spirit of Abdus Salam Award in 2018. Could you tell us about that moment and how you felt?
I’d only say that, in a very humble way, I really think I played a very small role in ICTP, in motivating young people and talking to them and just listening to them. And I’m talking about hundreds of people that I came across, and Aravinda is one of them. ICTP has a fantastic infrastructure. The secretaries are extremely efficient in every aspect. You know, at that time there was a publication office which, of course, changed because now we all type out our own papers. At that time, a typist was necessary. Some older people like Narasimhan would make use of it, a different generation. I myself just recently started writing my own paper. The librarians are all professional. You have a total sense of professionalism. And it makes the institution very good. So I’m very honoured to have received that prize. I think that I only contributed a little grain of salt to that beautiful place.
That’s very humble of you. Thank you so much for making time to talk to us so generously.
Interview with Lothar Göttsche

You have been with ICTP for almost three decades now. How did it all start?
What was the math department like when you joined, and how is it now, if you look back and see?
The student strength has always been about the same. At that time, there were 10 students in the diploma program that had started not too long before that. And it is still the case. What was a bit different then was that there were fewer faculty members. Most of the teaching was done with outside help, partly by visitors, and partly by faculty both from SISSA and from the university.
I am the coordinator of the diploma program now, and the teaching is mostly done by us as we have more inhouse faculty members. Earlier, there were more associates, more visitors and more postdocs, compared to the number of faculty members. The budget hasn’t altogether changed very much. Also, the emphasis may have been different back then. I mean, for instance, for the associates, and also for the postdocs to some extent, one would select people who we considered the best, also taking into account where they were working. As there were so few people, most of them would work basically independently. Maybe some of them could talk to us in our field, but there were many who were selected just based on how good they were; and they would just work by themselves. Now, there’s some emphasis, particularly for postdocs, that we normally prefer people who can talk to one of us. It has its advantages and its disadvantages, but this is the change that there is.
You said for the Mathematics Section, algebraic geometry was the focus area and it still remains so, but…
So this was in terms of the mathematical topics. The other aspect is focus areas in terms of ICTP vision, in terms of the outreach programs that you had or the diploma programs or associates program, has that changed over the years?
Another thing which was different is that there were quite a lot of funds from something called Sida. I think it’s a Swedish organization which at ICTP funded in particular the associates program, for people from the least developed countries e.g. Africa. And so there were many people selected for this. At some point that funding stopped, and now I think there are fewer associates from these countries. Anyway, this has changed things a little bit.
In terms of access to resources, the internet and online meetings have come up in a big way, especially in the past three to four years. But when you joined, how did you usually communicate with other mathematicians or access journals and books and how is it now?

So the building next door has nothing to do with SISSA now?
You have to now plan for a meeting where earlier you could just walk in.
Would you say your typical day has changed from when you joined to what it is now?
What do you think have been some successful events from the maths department during your tenure?
At a more personal level, there was one school which to me seemed particularly successful. It was originally the idea of Narasimhan, but finally I executed it based on his suggestion. It was called “Advanced School in Basic Algebraic Geometry”, a contradiction in terms. The schools organized are usually at a research level, and that means that you have these very advanced lectures where you also use advanced material and many things are just sketched, mostly with few details of the proof. So you don’t get so much into the nitty-gritty.

Talking about successful schools, there was this other school that comes to mind which was organized entirely online recently, during the Covid lockdowns. It was on commutative algebra and related topics. But the point is that this school was organized in a very different way from what we were accustomed to. Really using the fact that everything was over the internet. It was a three-month school, with a few lectures per week, exercise sessions, and some final exams, and over a long period of time with tutors.There were these lecturers from the US and from Italy and so on, and there were students from all over the world, but everybody stayed where they were. It was extremely well organized, and I think it was a very big success. There was an international team of organizers, but as far as I could see, the main driving force was Jugal Verma (from IIT Bombay). I don’t know if one could try such a thing once again. The normal thing would be to invite people here for three months, but if you cannot afford that, and if it’s a really big school in particular, we have shown that this kind of approach could work.
These schools that you mentioned, who typically comes to attend them? PhD students or postdocs?
And do the students have to take some kind of entrance test to be selected? What is the selection criteria?
You mentioned that earlier there were so few of you that you couldn’t really cover all of mathematics but now that you are more members in the Mathematics Section representing different fields, perhaps handling some of these tasks may have eased a bit than before?
There’s one colleague, who’s in some sense a half colleague, namely Pavel Putov. He is a joint member of the mathematics and the high energy physics groups, and what he does is not so very distant from what I do. I haven’t yet explored it very much, but there is some connection there, and I can understand what he’s talking about, though not all of it. Obviously, he has to try to express it in my language. Physicists are usually better at speaking both languages. What I do in algebraic geometry, like moduli spaces, has some connections to physics, so I do talk sometimes to the physics colleagues here. I used to talk to some extent with K.S. Narain, who was in high energy physics and is now retired. Then there was George Thompson, who has also retired. Now there is Bobby Acharya, with whom I occasionally talk. What he does is not so close to what I’m doing, but he often comes up with questions that I cannot answer most of the time, though sometimes I can. I got some inputs from Thompson and Narain, and there’s at least one paper that I wrote, which was more or less based on discussions with them. I have also occasionally had some chats with [Cumrun] Vafa, who comes here as a visitor. He is in high-energy physics, was originally from Iran, but he’s in Harvard now.
And then, there’s Don Zagier. What I do is somewhat related to modular forms and explicit calculations, so I had a number of discussions with him over the course of many years. I have only one paper with him, which I wrote before I came here, but we still discuss many things. I have personally known him for a long time because he was in the Max Planck [Institute for Mathematics] where I was also working.
In fact, we have carried out Don Zagier’s interview in Bhāvanā. Was that your first contact with Don at Max Planck or did you know him even before that?
I feel very indebted to Narasimhan. He brought me here
As you mentioned, there are a lot of visitors to ICTP every year. Any visits that you particularly remember, that you cherish?
My collaborations would usually start at conferences most of the time, either here or somewhere else. As far as I can see, the main purpose of conferences is that you talk to people and find some common interest. But for some reason with the schools organized here, most of the time you’re a bit too involved in the organization and worrying about this or that, or lecturing, not leaving much time to actually interact with people. There were certainly some interesting interactions, but I don’t think I initiated a collaboration here with visitors.
What are your future plans?
Are there any other thoughts or a message that you would like to share apart from these questions?
Thanks a lot Lothar for your time. Thank you very much.
Interview with Claudio Arezzo

First of all, thank you very much Claudio, for providing this opportunity to visit ICTP and talk to people working here in mathematics, and also for agreeing to this interview.
Thanks. To start with, perhaps you can tell us about your own journey in ICTP.
And then what happened around that time was wholly unexpected. You know, sometimes life seems like a succession of accidental occurrences. My predecessor here, a differential geometer and who was also a good friend of mine, decided to leave. And he casually mentioned this to me, encouraging me to apply. I said, okay, why not! And consequently, I joined ICTP as a research scientist in 2010, and I worked under the direction of T.R. Ramadas and Fernando Villegas – because it was a time when heads of sections were rotating. And I became the head of the section in 2018.
Speaking of your interactions with Indian mathematicians, we hear C.P. Ramanujam had some interactions with your family. Could you please elaborate?
Mathematicians used to visit us. Unfortunately, I don’t remember C.P. Ramanujam. I have a couple of pictures of him at my home with my older brother who actually remembers him better. That is because at that time Ramanujam had a very big and scary black beard. And my brother was then only four or five years old, and he remembers this guy with the big beard.
Unfortunately, I don’t have a picture with me. He was visiting Genova. Genova was a strong centre for algebraic geometry in the 70s through the 90s. And I think he spent quite some time in Genova and later on also became a good friend of my father.
Coming to ICTP, what are the changes that you have witnessed here during your tenure, especially in the last 15 years?

We at ICTP want to be a strong hub for people coming here to do research. So if you look at the programmes that we run, the schools that we run, the variety, the spectrum of topics that we try to cover – all of this is much larger right now, presumably than in the past. We conduct a lot of schools. Since the permanent faculty of ICTP is pretty small, we tend to be more open to people from outside to come and work here, and also if they happen to be working on topics that are not exactly our main expertise. I think this is happening everywhere. Of course, we talk to colleagues working in regular universities. There’s a lot of pressure about opening up to very new themes, and we have to be able to respond to such needs as well.
What are your future plans for the Mathematics Section at ICTP?
I remember talking to Narasimhan. He, of course, was a crucial figure, a very important figure for mathematics in Trieste; and I had the luck to talk to him many times. He was on our Scientific Council and he always made the point that mathematicians are very simple human beings, and have very little needs. They just need to have a few people to talk to, a blackboard and a piece of chalk and ideally, a good library. A good library helps a lot.
In fact, the needs are quite modest. Narasimhan made this point fairly often. If you want to start developing science in countries where the financial situation may not particularly be good, mathematics is an excellent point to start with because there is no need for big investments.
Since a part of your job involves a lot of administration duties, do you still find time to focus on your own research?
I discuss my ideas with these young people who have comparatively more time to develop the ideas further. And so I have managed to write what I think are quite interesting papers in collaborations with them.
And also the associates programme. I think it’s really a great idea. It’s unique. Perhaps one of its kind in the world. There are plenty of institutions which offer visiting fellowships, fortunately so, because it’s better than nothing. But the scheme of associateship at ICTP, I think, is really much better; for, the idea that every year we get to spend one full month together, discussing mathematics, makes the programme special. In fact, I think all my papers of the past five or six years were written either with postdocs or with associates. So, I’m using my position at ICTP to create new collaborations, to get in touch with new people. Perhaps maybe not as much as I would have liked, but still, this programme has enabled me to stay active as a mathematician.
While it is one thing to get other mathematicians to appreciate the work ICTP is doing, how could we convey the essence of the work done here to the general public?
So, from the technical point of view, it is true that it’s not easy to communicate what we do. Though personally I feel that mathematicians should make a concerted effort to improve this, because there are so many beautiful problems, beautiful lines of research, which are actually quite simple to explain to a lay audience. Then of course, you have to stay clear of the technicalities.
![The [first] 1992 batch of Diploma students. Seated (L to R): Stig Lundqvist, Abdus Salam, M.S. Narasimhan, Chidume and Alberto Verjovsky.](https://bhavana.org.in/wp-content/uploads/2025/04/ICTP-Diploma1992-1024x603.jpg)
For example, in my own case, I start from talking about soap bubbles and go on to eventually talk about black holes. That’s a classical talk that I usually give, because it’s part of my expertise. These are things that I actually study for my research. I always find the public to be excited by the fact that the geometry of a remote black hole is essentially the same thing as that of an everyday soap bubble – just slightly more complicated. But the truth, the honest truth, is that we know very little about that. And these are some things that I do, and you can play the same game in many other branches of mathematics. In essence, I think mathematicians should definitely make a much bigger effort to explain their work and its context to the general lay public.
In fact, for ICTP, what is also very important is to try to communicate these kinds of messages even to the policymakers. Of course, a big part of my job is to formalize agreements, sometimes with countries, sometimes with scientific institutions in the developing world. I need to convince policymakers of that country that it’s actually good to invest in science and mathematics, even in small amounts. In mathematics we don’t speak about millions and billions. Our requirements are more modest.
And then, in order to communicate the successes, I think there are two points regarding mathematics which are strong, and quite peculiar to mathematics. One is, by definition, the universal nature of mathematics. I think there is no other subject for which an Indian, a Japanese, a Brazilian, and an Italian can stay in one room, and after two minutes start talking about exactly the same thing, exactly the same problem. And I think this is fun. It’s beautiful. It’s probably one of the reasons why most of us have become mathematicians. In fact, I would say that about a half of them take up the subject for its sheer beauty, while the other half are drawn to the subject for this incredible feeling that you meet somebody completely different from your background, and in 30 seconds you feel that there is a perfect intellectual connection independent of what you think about everything else like, religion, culture, or food. So you can take this for granted, when you explain to the policymakers or to the general public as to why they should do mathematics. But we don’t do this enough, I strongly feel.
This above point, the universality of mathematics, is a critical point in our favour, also from the conceptual point of view. Mathematics is the language of all science, and everybody should understand some deep mathematics, whatever type of science they want to do. This message should go through very clearly both to the public and to policymakers.

And so the successes are actually faster than in other subjects. You really don’t need to solve any other problem. You only have the mathematical problem that you are studying. And in fact, the breadth of the connections that you can build is even bigger, because you see, if you are doing experimental physics, there are probably more than half of the countries of the world that cannot afford doing serious experimental physics. They simply have no access, because it’s too expensive. While for mathematics, when we show the pictures of where our associates come from, where our postdocs come from, where our diploma students come from, you really feel that the whole world is participating.
A certain country may be particularly poor, while certain other countries could be a bit better in terms of their respective economies, but for mathematics per se, this fact has very less bearing on the quality of its output than what you would expect.
The other strong point in favour of mathematics, which is also true for theoretical physics, and so we are in line with the core spirit of ICTP, is the possibility of open access to resources; this is where in principle things could become complicated for everybody. Journals are very expensive, books are very expensive, but fortunately the community of mathematicians have actually built ways to provide open access essentially to large parts of the community itself. There is certainly still a lot to do, but it was not like this in the 80s at all. I remembered again, when I was talking recently with my predecessors, that providing access to books was one of the main things that ICTP was doing in the early days. Fortunately, this is much easier now. Our library now, for example, electronically provides access to all electronic books and journals to all our associates. I mean, you don’t have to come to Trieste to actually read the book.
Do you recollect any special moments or things that you cherish at ICTP? Any anecdotes?
Of course, for a mathematician, the greatest achievement is to prove a great theorem. But this is something you could have done in any university, in any place. Specifically as ICTP faculty, the greatest achievement, and actually I would say it really gets almost becoming an emotional feeling, is when we see the success of some of our affiliates, say our diploma students; when we realize that after five intense years they have transformed themselves into important mathematicians. This is the greatest success in my opinion, and one which fortunately happens quite regularly.
Just a couple of months ago, one of our former diploma students, Soheyla Feyzbakhsh, won the Veblen Prize in Geometry, which is the highest recognition in the mathematical community for geometry. She’s an Iranian woman who was here at ICTP, and then went to Edinburgh for her PhD. She has always been in touch with us, and has visited us at Trieste afterwards too. She’s still very young, but has already won the Dubrovin Medal, and the Veblen Prize. This is very satisfying.
Another famous case is of Moustapha Fall from Senegal. He was a diploma student here at ICTP, and who continues to be a friend of ICTP. After a few years in Germany, he decided to go back to Senegal. We are now working together, both mathematically and institutionally to improve the state of mathematics of Senegal. Fall was an invited speaker at the International Congress of Mathematics, and has won the Ramanujan Prize for Young Mathematicians. Since we both work in the same area of research, I also understand his work, and I think he is doing really beautiful research.

Often enough, it’s our associates who receive very important recognitions. I mean, our associates actually go on to become faculty members in prominent universities, and sometimes they are already quite well established. To know that they have been recognized with some important prizes, or that they actually proved some important theorems, independently of us, that’s really the best feeling. I mean, these are really true moments of happiness for us.
Is there a favourite mathematician for you – someone who you looked up to? Maybe your father?
I guess you tend to have this kind of little pantheon when you are younger, and when you are a student. And I too was really looking at the older generation of mathematicians, like Kunihiro Kodaira, John Milnor, and Phillip Griffiths, for example, as my heroes in mathematics. Going back a bit further, I have always considered Gauss and Poincaré as my all time heroes.
Actually, I remember the first time I talked to Misha Gromov and Sir Michael Atiyah. They were really big emotional moments. I was a graduate student then, and did not understand much of what they said, but just the fact that I was talking to them was special.
Thereafter, I started specializing in my area. And in my area, there are four singular figures who have shaped the research of the past 40 years. They are Shing-Tung Yau, Simon Donaldson, Richard Schoen and Gang Tian, for sure the most influential and deepest, complex differential geometers and geometric analysts. I’m very happy that Yau, Schoen and Tian have visited the ICTP frequently in various important capacities. I’ve not succeeded yet in getting Donaldson to ICTP.
And also I must mention this fact that when I went to do my PhD at Warwick, James Eells had then only recently retired. Eells had worked with Alberto and M.S. Narasimhan, and it was the three of them who actually created the Mathematics Section of ICTP. Of course, at that time in Warwick I was unaware of ICTP and its history, but Eells was sort of the grandfatherly figure to every graduate student there. He would come to the common room, have tea with us, ask what we were doing, and give us solid advice. He was incredibly warm, very funny. Typically, mathematicians tend to be very serious. We tend to prefer to be a bit boring rather than come across as being stupid. He was very lively and very engaging in conversations, always looking at the positive side of things, all besides being a very deep mathematician. He was a magnetic personality. I mean, if you had been to even one of his seminars you would have seen it yourself. Unfortunately, he passed away before YouTube became available. His seminars were simply unforgettable.
So there is no public recording of his seminar, as far as you know?
Would you like to give any general message to younger students or to people who aspire to be mathematicians?
to put mathematics in the much bigger picture of human attempts to derive joy from beauty of thought, art and nature
Mathematics is essentially a subject where we know almost nothing, and almost any interesting question is open or unresolved, and that is what makes it very exciting to pursue. In some sense, this realization also makes life a bit stressful, because you realize that you know almost nothing, and you have to live with this perception that we don’t know what we would like to know, which is a bit disturbing, but at the same time, it’s very exciting, because everything is still to be discovered.
In terms of practical life, I connect with what we said before, which is – the universality of mathematics. This makes the everyday life of a mathematician very non-routine, especially in the eyes of normal people. One moment I’m chatting with two friends in India, and then immediately later, I have a meeting with a friend in Vietnam, and then there is one collaborator from the US who would like to talk to me. So it’s also an exciting life in these terms, where you get to know people from completely different backgrounds. And at the same time I want to be honest here. You may also entertain a little feeling of being useless. Suppose that you have solved the Hodge conjecture, you become famous, you win the Fields Medal, and you also get the one million dollar prize money. Then what?
The real interest for me is more in the history of the problem
What have you changed in the world? Because you look at the world, and you are not happy with how the world is going. Would the proof of the Hodge conjecture – change anything? Presumably not, or possibly infinitesimally maybe. Of course results of this level are significant achievements for all humanity, we know that; and I do believe devoting much of our energy to make progress on these is a beautiful and satisfactory way to spend our intellectual life. But I also sympathize with a feeling that seems now to be growing in the younger generations about doing something which has more direct application in real life.
I sympathize a little bit less about doing this, because you could then gain a lot of money. I prefer to think of people who want to do something applied because they can actually have a bigger grip on the world. Still, I think mathematics, and also theoretical physics, the blend of subjects available to study at ICTP is superb because I believe that a solid foundation in mathematics and theoretical physics is still the best way to achieve this. So, if I could suggest something really to my own kids, it would be to study mathematics, theoretical physics or computer science, but from a very fundamental point of view. If you want to study mathematics for economics or artificial intelligence, and try to improve the world, for which there is a huge demand, then go ahead. Don’t just think about earning a five million dollars per year of salary. Actually, the younger you are the more difficult things you should study and then you can decide later, maybe when you need to enter a PhD school. Actually, you could even take a five year course in mathematics and theoretical physics, and then go on to earn a PhD in artificial intelligence or economics, if you want.
But, there would be a version of this advice for policymakers too. All this seems like putting pressure, especially on the developing countries. But I can tell you that the developed countries too are exactly under the same pressure. We want our countries to be the leaders in new revolutionary technologies. Unfortunately, there is no royal path to geometry, as Euclid said to the king of Egypt. What he meant is that the idea that you can start right away and, in two years, you will have results is rubbish. I think this only creates a new form of colonialism. It’s yet another version of our old enemy. If you are not prepared with solid foundations, the only thing you can become is just a tool of one of these big multinational companies, well paid only for some time.
On a lighter note, do your kids also not want to do mathematics, because you do mathematics?
When you are mentioning about people not being interested in mathematics or thinking of it as too difficult, do you get the sense that maybe some problems in number theory are easier for the layman to grasp, like Fermat’s last theorem or Goldbach conjecture which are easy to explain to a school kid also, but which may not be true for your or other disciplines of mathematics; so in that sense, do you feel a bigger challenge to kind of explain these things or to get students motivated in these domains?
The real interest for me is more in the history of the problem. A great many very clever people have tried, they didn’t succeed, and so you see the challenge. But the fact remains that deep within, I am a geometer. I really feel this has very little to do with my own perception, and my own personal experience. I would really like to solve a problem in mathematics that actually has to do with nature in some tangible form. So, for me, for example, finding a new solution of the Einstein equations is perhaps a more beautiful mathematical problem. And if we can find it, what will it look like? What is its curvature? How does the curvature behave when you approach the black hole(s)? And how multiple black holes deeply interact, even “just” geometrically? These are, to me personally, what I consider beautiful mathematical problems. They are almost directly inspired by nature.
And on similar lines, what really happens when two soap bubbles touch each other? What is the dynamics of the membranes where two membranes touch each other? So, I’m really a geometric analyst. Coming from that perspective, the fact that a strange polynomial has an integral solution doesn’t really warm my heart. But I fully respect it and everybody should be well aware of the importance of building a universal language, apart from attacking and solving a specific problem. The magic and beauty of maths is really about this. History of science teaches we have to do both, and at ICTP we do our best to support all these aspects.
Thank you so much Claudio for your time and your insights.
Interview with Stefano Luzzatto

We understand you moved from Imperial College London to ICTP. What motivated this transition?
After my PhD, my wife at the time, who is British, moved to the UK to start a PhD herself and I followed. I took up a postdoc at Warwick and then a permanent position at Imperial College London. My daughters were born in the UK, but we always liked Trieste and I had a deep appreciation for ICTP’s mission. In 2008, a position in my field opened up at ICTP. Many people thought I was taking a risk by leaving Imperial, but I discussed it with my wife and she was very enthusiastic, and I was drawn to both Trieste and ICTP’s mission, so I applied, and got the job.
Since you did your PhD at SISSA, could you tell us about the collaboration between SISSA and ICTP?
Apart from SISSA, are there structured collaborations with other institutes?

We also of course have many collaborations with institutions in developing countries, through many of the ICTP programs. These collaborations can include jointly organizing schools and workshops, hosting short and long term visits of scientists to ICTP, and many other interactions. One particularly interesting program is the Affiliated Centre program which allows us to work particularly closely with one institution over a number of years to support its efforts to become a regional centre of excellence in research and training.
What is the state of high level mathematical research in different countries?

Why are so many countries still lagging so far behind?
It should also be said, however, that these days mathematicians everywhere, at least the more senior mathematicians, are less isolated than they were before, with more access to scientific literature and events, both online and in presence, and to the resources needed for international collaborations. At the same time another important factor has intervened which is having a hugely counterproductive effect, which is that many governments and institutions are putting huge pressure on researchers to publish large numbers of papers but, unfortunately, without paying much attention to the quality of the research. Some formal quality checks are often required but these are generally very mild (for example to publish in so-called Q1 journals, but there are a huge number of Q1 journals with a huge variation of quality). While these policies are often put in place with the best intentions of promoting research, the unintended effect is essentially an encouragement to publish a large amount of low-level research, since there are really very few mathematicians who can publish a large amount of high level research. I personally think that this is the elephant-in-the-room of all discussions about capacity building and needs to be addressed explicitly if anything substantial is going to change.
What about the International Mathematics Master (IMM) program you have been involved in?
There are several ideas which underpin the philosophy and the practical implementation of the IMM and it’s not easy to summarize them all in a few sentences. One key point is that every country, even those with a very poor educational infrastructure, have some exceptionally talented students who are creative and have personal initiative and great potential. The goal of the IMM is to give these students the opportunity to learn from the best international researchers so that they can themselves become excellent researchers working at an international level and become research leaders and supervisors of students in their own countries.
This is of course not at all a new idea. Many other programs, even programs run by national governments, have set themselves the same goals, usually by offering scholarships to study in international institutions. While this approach has undoubtedly been very appropriate for many students, and led to a number of success stories, it does not seem to have ultimately had a significant impact on capacity building in most countries. Some people would argue that this is due to “brain drain”, in other words to the fact that these researchers do not return to their home countries, but I don’t think that is the case. If it were, we would see much more diversity amongst the researchers and faculty in European and US universities. I have not done any systematic analysis of the data, but my own impression is that there are instead two main problems. The first is that most students who win these scholarships face a huge culture shock, not only personally but also academically, in terms of teaching styles and going from being top of their class to suddenly being surrounded by students who have much stronger background than them. Therefore, many of them in the end are not able to successfully complete their training. The second is that there is often no coordination in terms of what these students study, so those who actually manage to complete a good PhD and return to their home countries are isolated and there is no “critical mass” in their research area, making it much more difficult for them to do good level research.
The core principle of the IMM is to avoid these issues by bringing the international faculty to the students rather than the other way round. It is a full two-year MSc program which is hosted by a local university and where each course is taught by a team of 1 local and 2 international faculty. Each international professor goes for two weeks during which they teach their part of the course more intensively. We’ve had to design a new teaching model for this but it seems to be working very well and has a lot of benefits, not only for the students. Indeed, it leads to the regular presence of a large number of prestigious international researchers in the host institute. During their visits they can give seminars and interact with the local faculty, possibly starting some research collaborations, thus making it almost an international research centre.
One thing I want to mention is that the response to our call for interested international faculty has been overwhelming. Many more faculty have expressed their willingness to teach in the IMM than needed, many of them very well-known researchers working in prestigious universities. And it is worth emphasizing that while their expenses are of course covered, they are not paid, and thus are essentially donating two weeks of their precious time to the students in the program. It is clear that there is an essentially unlimited number of potential volunteers and this is a key to the feasibility and scalability of the program.
Which countries currently host IMM programs?
In September 2024, a new IMM was established at the National Higher School of Mathematics (NHSM) in Algeria, again one of the very best universities in the country, by the will of the Director of NHSM Prof Ahmed Medeghri and the IMM local coordinator Prof Rezki Chemlal. In January 2025, there was a formal IMM inauguration ceremony with the personal participation of the Algerian minister of Research and Higher Education, Prof Badel Kammari, who has expressed full confidence and support for the IMM model.
Several other universities in other countries have also expressed an interest in hosting an IMM program and discussions are ongoing. The IMM model is very flexible and can be easily adapted to different local contexts while maintaining the core features which characterize it. The idea is for it to grow into an interconnected network of universities which will contribute even more to its international dimension.
Jacob Palis is a Brazilian mathematician who has had a major influence on mathematical research in Brazil
What are the other challenges you have had to encounter here? Do you have to deal with the governments, or do you just deal with the university which takes care of the rest? Also, how do you identify which university to work with?
Universities which want to host an IMM program need therefore to show that they can cover the costs and that they have suitable infrastructure for hosting the classes and accommodation for students and visiting professors. At first sight this may seem to be challenging but in fact it is an extremely cost-effective model and gives any university the possibility of having a world-class MSc program taught by world-class professors at a fraction of the cost of hiring even one or two of these professors on a full-time basis.
Could you tell us about some mathematicians with whom you have collaborated, and who have made an impact on ICTP’s programs?
Before your PhD you did an MSc in Philosophy of Mathematics Education. Could you tell us something about that phase of your student life?
The mathematics they needed to learn was really simple high school mathematics and I was completely baffled by how such creative, intelligent, and capable students could be struggling so much with it. I started trying to understand why this could be and ended up writing an MSc thesis arguing for the importance of distinguishing between the dialectical logic that the mind uses to understand mathematical ideas and the formal logic that we use to articulate and formalize these ideas. I even published a short paper on this topic in a philosophy of mathematics journal, although re-reading it I’m a bit embarrassed by my “youthful enthusiasm”.
Has this influenced your later teaching?

I have been using this method for the last few years and the feedback from the students has been very positive. Sometimes in class all the students are split into small groups and working and the atmosphere is so much of focus and concentration, it’s really quite amazing. I sometimes tell them it’s time to take a break and they completely ignore me.
Yoccoz died prematurely, leaving his personal mathematical library as a donation to ICTP, to eventually go to an institution in Africa.
Coming back to ICTP, especially in your field do you feel a need sometimes to talk to people in physics or people who are studying real world phenomena and then work out how ideas from your field relate to those situations? For instance, to understand the interplay of order and chaos in various physical systems?
One very interesting direction of research which bridges, to some extent, this gap is that of “computer-assisted proofs” using “rigorous numerics”. This generally combines deep analytic and theoretical arguments with non-trivial computations which are however limited to certain kinds of estimates for which one can obtain rigorous error bounds. I have some limited experience in this direction, mainly in relation to the study of the famous quadratic one-dimensional family of maps f(x) = x^2+c. This family exhibits extremely complicated dynamics which moreover depends very sensitively on the parameter c. It is known that for an open and dense set of parameters it has so-called “periodic” dynamics but the complement of this open and dense set (which is therefore nowhere dense) has positive Lebesgue measure and almost every parameter in this complement exhibits so-called “stochastic” dynamics. However, given a generic parameter we do not at the moment know how to tell whether it is periodic or stochastic and nor do we even know the actual measures of these two sets of parameters. With a number of co-authors I have been working for many years on developing some computer-assisted arguments to address this problem.
Over the course of your association with ICTP, what have you felt has changed? Both in general with ICTP and in particular with the maths department.
Any memories from your meetings with mathematicians here or any adventures you have had in ICTP that you will tell your grandchildren?
Since I’ve been here as a researcher and faculty member, I have felt absolutely privileged to be able to contribute to the mission of ICTP. I have been to so many different countries and interacted with so many talented and determined students and it is amazing to feel that I am, hopefully, making a difference for them. I have supervised PhD students from Senegal, Nigeria, Turkey, Uzbekistan, Pakistan, Indonesia. Each one has brought so much passion and curiosity to their studies and it is a pleasure to see them going into the world and becoming independent researchers.
I have felt absolutely privileged to be able to contribute to the mission of ICTP
As we wind up this fascinating exchange, is there anything that you would like to share with us and with the rest of the mathematical community?
The second is also obvious but maybe not often so recognized. Apart from a few exceptional cases in which mathematicians can get very competitive, I would say that on the whole it is an extremely generous community. By far most mathematicians love to share their thoughts and discuss and collaborate and encourage younger researchers and suggest problems to work on. I think this explains for example why so many researchers volunteer to teach in the IMM program. It is so reassuring and comforting to feel that you are surrounded by people who may sometimes be somewhat shy or introverted but are nevertheless generally, deep down, very kind-hearted.
Thank you and best wishes to your wonderful magazine Bhāvanā.
Interview with Emanuel Carneiro

Thank you for making time for us. We would like to hear about your background before coming to ICTP, and how your association with ICTP got started.
So I came back to Brazil. I had one year experience outside the academic environment. I worked for a consulting company in Rio de Janeiro and São Paulo. It was a very nice experience too. And then one year thereafter, we’re talking about 2011, I decided to go back to academia. So I joined the faculty at IMPA, the Institute for Pure and Applied Mathematics. It’s one of the nicest institutes in Latin America, and is located in the city of Rio de Janeiro, in the neighbourhood of the Botanical Garden. I worked there from 2011 to 2018, first as an assistant professor and then as associate professor.
But around 2015, I came to know about ICTP from one of my professors in Austin, Fernando Rodriguez Villegas who, as you might know, was the head of the Mathematics Section here. He told me about the associates program. Coming from Brazil, I was eligible to apply and I applied to the associates program. So I got to become an associate, a Simon’s associate, here at ICTP. And in that time, I came to visit ICTP in 2016 for two months and then again in 2017 for two months. This is how I came to know ICTP and thus started my relationship.
It was a very nice experience. I would come here, usually in the fall, around this time that we’re talking now, September to November. And I would bring students, talk to my collaborators here. Some collaborators would come from Europe to visit. It was a very nice time to be away from your, let’s say, usual day-to-day duties that we all have in our universities – administration, teaching, and so on. It was a time that you could concentrate on research here. In 2018, when they opened a permanent position here in the section, I ended up applying and I got offered the post here. This is how I moved. It was a nice opportunity and we, my wife and I, decided to move. It was a good moment as we had just had children. Rio de Janeiro had become a little bit of a big city for us, and we always had this idea to live in a smaller place. And this opportunity presented itself. So we decided to give it a try. We came here in 2018. So we have been here for almost six years now. It’s been a lovely experience. I’m still very much in touch with Brazil, with my institute that I worked there before coming here. You know, it’s part of the job here to actually keep promoting and interacting with my friends and my fellow collaborators from the southern hemisphere, and I go there as much as I can.
So you rent an apartment here, or do you stay on campus?
We saw one photograph of you on your webpage with your kid.

Given that it’s a small group of permanent scientists spread out over 5 sub-departments within the maths section, is there much opportunity for discussion with your peers?
And we have mathematics, pure mathematics to be precise. Out of these six that I described, mathematics was the third one to come up. The institute started with high energy physics, which was Abdus Salam’s main field. Then condensed matter, and then mathematics since 1986. Now in ICTP as a whole, we might be about 45 faculty members. In mathematics, we are seven. Perhaps I should say eight, because we have two faculty members in the maths section, who are, let’s say, joint appointments.
So depending on one’s count, we are seven or eight in mathematics, which means that we are kind of 20% of ICTP. Talking about the Mathematics Section, we are small. And the idea is not that we aim to be a group of eight people working in the same field. The ideal configuration here is that you have each of the eight representing one field of mathematics. It has advantages and disadvantages. The obvious disadvantage is that among my peers here in the section, I don’t have anyone that works directly in my field. Perhaps one can also see it as an advantage because I can talk and interact with people from different fields. In my personal perspective, I believe that some of the most beautiful pieces in mathematics these days come from the plurality of fields. You see a person from analysis talking to a person in differential geometry, or an analysis person talking to a number theorist and so on. I like to think of myself as being a bit dynamic and willing. I’m always in favour of collaboration.
Another of the advantages is that each of us gets to manage or take the lead on a small group of people that come here – your visitors, the associates, the collaborators, and the students. So each of us here have a smaller group of people that are kind of connected to you. Let’s call it your research group. We just had lunch with a postdoc, two students, and we are a small group, but we talk together, we work together, and we organize our seminars. So I feel very comfortable here with this modus operandi, which suits me personally. It’s a good size of a group that I have, a group of four or five people. More than that, it would be difficult for me to manage. I regard them as people working with me. I can work with four or five people together, as I’m a very collaborative person. Most of my papers are written in collaboration. I have published with all of my PhD students. I like to talk and discuss mathematics, and I believe this is a great way to make friends. This makes mathematics, which is a difficult subject, a bit less difficult, right?
Coming to my subject, I work mainly in analysis, more in harmonic analysis, with bits of applications to other fields like analytic number theory, a bit of approximation theory, and bits of PDE (partial differential equations). The main part of my research over the past years has been mainly harmonic analysis and analytic number theory. We have a postdoc from India who works in analytic number theory. It’s nice that people with different backgrounds get to talk and find a common ground. And a person that is better trained in one field can pass on that knowledge to the other.
I think there is a lot of opportunity here for discussion because ICTP is much more than just the faculty members. It has postdocs, a very big group of associates, like I was before. Being an associate of ICTP means the following. You get a fellowship for five years usually, five or six years, by which you get to come to ICTP every year for a month or two. We have about 250 associates in the whole institute. In mathematics, we get about 50 associates who are distributed in different fields. Geographically, they all come from countries in the global south, and they visit in different periods of the year. We now have one associate that is a collaborator of mine from Argentina, sitting right next door. At any given point in time, our group will be me, a postdoc, two students, and one associate. So we always have a group of five or six to talk to. There are also visitors. The Associates is a program targeted just for developing countries, but we do have visitors from Europe, from the United States, from Canada and so on. They come with a different source of funding. So whenever I want to invite a collaborator of mine to give a seminar, we can do that too. Most of the mission of ICTP is, of course, to promote science for the scientists working in developing countries. But we cannot be oblivious to the fact that it is part of the mission to also put scientists in contact with colleagues from Europe and North America because this is how we all grow, right?
Is there a need or opportunity to interact with other (non-Mathematics) sections within ICTP?
I have had some connections with mathematical physics in the past, but mathematical physics is a broad name I guess. Mathematical physics that I’ve been interacting with in the past, a few years ago, was mainly with the Boltzmann equation and the study of gas, which is not the type of things that our colleagues in physics here do. So I haven’t had the chance to, let’s say, collaborate on a specific project with any of the colleagues from physics here, yet. Someone like Don Zagier would be very good at interacting with physicists, because for certain problems, physicists may have a better intuition than mathematicians. We sometimes can help with formalizing stuff, ideas that are not particularly well developed, but have a nice intuition. Don Zagier is an expert on these sorts of things.
What changes have you seen at ICTP over the course of your tenure here, both in general and specifically in the context of mathematics? For example, how have things changed during and after COVID?

|
 |
| The neat and pleasant Mathematics Section corridor maintained by the dedicated housekeeping staff ICTP Photo Archive | |

About the changes I’ve seen, we are adapting to the changes that the outside world brings on us. Scenarios like inflation or costs of air flights which have risen in the whole world, are affecting us a little bit. In the past, we would have a conference here, and bring 40 people from all over the world to come here. Now to do the same thing, it costs five times more, and our budget didn’t increase by five times. So we had to rethink the model. We have to be a little bit more creative and say, instead of organizing a conference here and bringing 40 people over, we could switch to a hybrid mode. Or if we’re going to bring 40 people over here, let’s not make it one week because it’s going to be not very cost-effective. So let’s bring them here for three weeks at least, or a smaller group for more time. I think this is more effective than doing large events. It aligns with lots of things that we’re seeing in the world today, to diminish this carbon footprint for example. These changes are happening at ICTP, as they are happening everywhere else.
What have been the major achievements of the math department at ICTP from your perspective?
We have programs here at ICTP to help the career of a scientist at all levels. Our first program is a diploma program. We have 10 students every year from developing countries that come here for one year. This at a master’s level. Since we’re talking of developing countries, the students typically don’t come from Brazil or India or Argentina or China. One can get a good master’s education in India or a good PhD in Brazil. The students who come here are generally from Africa, Middle East, and Central America. So the 10 students who come here, stay for one year with us and go through our program. The objective is that, after the diploma, they will be in a position to apply for good PhDs or masters elsewhere. And most of them succeed, and go to competent places for PhD. This is the first educational program that we have here.
And then we have a PhD program in collaboration with SISSA. Two of my students that we met here are PhD students at SISSA. Besides the PhD, we have a postdoctoral program. Since we are eight members in the section, we usually have eight to ten postdocs at any point in time, with a good priority for postdocs coming from developing countries. It doesn’t mean that we cannot have a postdoc from Norway or Sweden. But out of our ten postdocs, at most one or two, if there are that many, will not be from the developing countries. Then we have the associates programme I already told you about. The associates can be junior, regular, or senior. We have all of these programs going together, which makes ICTP a kind of place to help the career of a mathematician to advance from the very beginning. And to me, the major achievement of this section is to have successfully carried this over the past several years. And still serve as a lighthouse, a point of focus for these people to come back. Some of these people later give back, sending their students here, which helps revitalize these programmes.
So what is the measure of success from an ICTP perspective? You provide the training and then after that, do you have certain metrics that you use to rate yourselves?
We have programmes here at ICTP to help the career of a scientist at all levels
And the whole ICTP organizes about 50 events every year. We have metrics and data for the participants and we try to analyse the number of participants, gender balance and such, and we try to improve. We try to improve the geographic balance. Now we’re trying to have projects to improve the participation of scientists with families. If you are bringing your kid here, we’re thinking of making a child care facility here. These are kind of more, let’s say, fact-based metrics that we can think of.
So as such, the number of seminars that we organize, the number of events, PhD students, and such things have to be looked at, not on an yearly basis, but maybe in a span of over five years. Maybe the number of PhD students who are graduating. We can then feel a measure of success or a feeling of satisfaction when we see some of these cases becoming successful later elsewhere. We have had the example of Mouhamed Moustapha Fall, who was a diploma student here who went back to Africa and works there presently. He won the 2022 ICTP Ramanujan Prize. Then we have a former diploma student, Soheyla Feyzbakhsh, who is now a professor in the United Kingdom. She received the Boris Dubrovin Medal in 2024, an important prize from SISSA. She’s from the Middle East, I think, from Iran. There was another postdoc here, Jose Madrid from Honduras, who is now a professor at Virginia Tech in the United States. So you see, you have lots of these cases of successes. We have to be careful working with metrics in mathematics. Some countries are going in the direction of counting papers, counting citations to evaluate people and so on, which I particularly disagree with. I believe this is very dangerous and this is not the right way to do these things.
It’s quality, rather than quantity metrics….
Instead of doing massive things for a massive number of students, I prefer to do smaller things for a smaller group with more attention and more focus, because I believe in the multiplicative power that this has.
Can you tell us about the visits and interactions with mathematicians at ICTP that you cherish the most and which you feel have contributed significantly?
But if I had to choose one example for you, of a mathematical interaction which kind of aligns with my own philosophy and the mission of ICTP, there was a moment two years ago where we had our permanent group here – two postdocs, one student and me. And there were two associates visiting and another visitor who just happened to be visiting. So we were a group of seven working in my field. Seven people of different ages and seniority. Two postdocs, one student, four professors. All from Latin America. It was nice to have these seven people interacting and talking to each other. One of them would ask me, “Emanuel, can we talk at 3 o’clock?” And I would reply, “no, I cannot talk at 3, but can we talk at 4 instead?” And then he would say, “no, at 4 I’m going to talk to the other guy”. This thing went on. And at some point I realized that this group is a bit bigger than what I usually handle. So I told them, “guys, why don’t we get together in a room, the seven of us, and we all propose problems that we like to talk about. We have two months. Each of us propose something and maybe we can converge on a project that we can all work together for the next two months”. So we went to a room, talked and then at the end we picked one problem. It turned out to be a problem that I had proposed, something that I had thought about for several years before and made no progress. Maybe you can give me some new ideas, I said. And suddenly one idea came through and we made a little progress. Then came another idea and we made a little bit more progress. At the end of the day, for two months, there were no more appointments. Each one of us was thinking and talking about the same problem. Finally, we ended up working together and writing a paper with seven authors, a nice 40-page paper that is now submitted for publication in a nice journal. I was very proud to have accomplished this, let’s say, South American project. Mathematicians from developing countries working together for two months in different capacities at ICTP. It could represent the philosophy of what this place is about. This is my example. I can send you the paper later.
Some countries are going in the direction of counting papers, counting citations to evaluate people, which I particularly disagree with
Coming from Brazil, do you see ICTP having an impact on the development of mathematics and mathematicians in Brazil, and of any ideas on how much more could be done?
I have represented ICTP in the Brazilian embassy in Rome, so it’s really science and diplomacy together
Could you tell us about your plans and vision for ICTP?
To collaborate with others, some of the other countries are perhaps now at a stage that these countries were thirty or forty years ago. It’s now more of a sense of community. Everybody is trying to help each other. Plus we have now the fact that some countries are at war, so ICTP remains a beacon of peaceful interactions. They have here scientists from Ukraine working with scientists from Russia, scientists from Palestine working with scientists from Israel and so on. At ICTP, as you enter this building, you see no borders. Everybody is welcome to do science. My singular vision, of course, is to keep this going. Personally, if I had to choose between quality and quantity, I would certainly be inclined towards quality. I would prefer a little bit less of activities, and foster more focused, more targeted programmes, giving more attention, more time to a slightly less number of people, especially since everybody has access to the information now. I believe in the multiplicative power of science. But this is just my personal view of things. Of course, I know that when we defend ICTP in the international scenario, to get the funding, it’s always important to say ICTP has 6,000 visitors per year.
Other faculty members may have different experiences and perceptions. Everybody has their own predilection. What makes this place special is actually the union of all of these things. One person can complement the other. I think we’re doing great. And I expect it to keep going with the same trend. Part of the success of ICTP is represented in our countries. ICTP is what it is only because people coming from various countries respect the centre, and look at it as a point of reference, a place where they are welcome whenever they want to come. While I always publicize our opportunities here, I also tell them that these things are often competitive and maybe one doesn’t win this year. But if you’re ever around or if you have funds from your country, you’re always welcome to come for a visit. Even if you’re visiting Trieste for tourist reasons, if you want to stop by, you’re always welcome. Every scientist working in developing countries or scientists in general, are always welcome here. This is what’s magical about this place.
At ICTP, as you enter this building, you see no borders
You said that when you came here first as an associate for a couple of months, that it was a nice break from the typical university kind of duties you had with classes, administration and so on. So is there a typical day as such at ICTP that you have, or is every day different?
It’s part of our job to try to combine the best of both worlds. To do our own research and to serve as an example and inspire and try to help as much as possible. I think all of us in the Mathematics Section have this disposition. This is also something very nice here. Everybody in the section is very nice and friendly.
We were just speaking to a student here from Uzbekistan and he said that it was another peer of his through whom he came to know of ICTP. You said you came to know of ICTP because a professor of yours told you about it. So is word of mouth how awareness about ICTP is growing, or are there public awareness programs that are done?
We have each of us doing our own “spreading”. I know the Brazilian Mathematical Society mailing list, I go there and put it; somebody else knows China, and somebody else knows India. We have a YouTube channel where the lectures and courses are posted. We publicize these things in conferences. So we have several channels to spread information about ICTP. Word of mouth is certainly very important to me.
Thanks a lot for your time and sharing your valuable insights about ICTP.
ICTP and Trieste: An enduring association
– A postscript by C.S. Aravinda

The only qualification for me to be writing this postscript seems to be my 36 years long association with the Mathematics Section of ICTP as a 9 times visitor, starting from my first visit in 1989 to date, with an average frequency of once in four years. My reminiscence, therefore, intertwines with certain aspects of my academic journey, focusing on the sheltering role of ICTP in my evolution as a mathematician.
My first visit in 1989 was as a participant in a two-week workshop called “Topical meeting on hyperbolic geometry and ergodic theory”. I was a graduate student at TIFR, Mumbai then, and it was my first ever journey by flight, let alone being my first trip outside India. The excitement was at its peak in all aspects.
I had, of course, heard of ICTP earlier. More than that, one of my senior colleagues and a fellow differential geometer, Ravi Aithal, who had worked in the area of “harmonic maps” had spent a year at ICTP as a postdoc. I had thus heard of Jim Eells – a name synonymous with harmonic maps.
Seeing Jim Eells at ICTP on the first day of the workshop, speaking at the ceremonial opening as the head of the maths section, was a treat to watch. Quite simply, I felt `he doesn’t speak, but performs’; the exuberance mentioned by David Elworthy, Claudio Arezzo and others in their recollections, was in action. It was a precursor to the next two exhilarating weeks. One lecture course that stood out for me personally, for its palpable relevance to the problem I was working on, was that of Anthony Manning. I remember talking to him at the end of a lecture for a technical clarification, and coming out feeling jubilant at a new understanding gained.

I must record here that my physicist friend Sunil Mukhi, who was a postdoc at ICTP before joining TIFR as a faculty member, had prepared me well enough to explore the beautiful town of Trieste. He was a great motivation in another aspect as well, namely to appreciate the musical sound of the Italian language. All in all, my first visit was truly enriching – an experience of a lifetime.
Back home, and with new found energy, I had made just a little but a definite progress in my thesis problem. With my PhD scholarship ending in October 1990, and my advisor Dani’s imminent long stint away on sabbatical leave around then, he suggested that I could go to ICTP for a longer position. I couldn’t be happier. His letter of support working the magic, I was back again at ICTP in January 1991, and I set myself to work in earnest on the problem. That year coincided with M.S. Narasimhan’s year-long sojourn there, providing me with a rare opportunity to interact closely with him. I got to hear from him about his early days at TIFR, K. Chandrasekharan’s visionary planning of the activities of the School of Mathematics, and about the people he admired. Cricket and Carnatic music were two other things of common interest that we enjoyed talking about.
The other long-term residents of the maths section were warm and lively, and we bonded well like a family, with the dynamic personality of Alberto Verjovsky introducing each one of us to the other, and creating a wholesome positive ambience. The two secretaries – Sharon and Alessandra – were exemplary in their warm disposition to the constant flux of short and long term visitors, and the air was always upbeat.
It was one day in early 1991 that Alberto introduced to me a newly joined postdoc, namely Alexander Reznikov, as a fellow differential geometer. It was only then that I learnt that Sasha is a commonly used short address for Alexander – thus slowly beginning to appreciate nuances of different cultures, especially in that remarkable European continental setting of Trieste with the variation of languages and dialects in its vicinity, akin to an even larger variation in the familiar Indian subcontinent.

The other distinct memory from 1991 is the dynamical systems workshop sometime in summer. The maths section corridor was literally abuzz with activity and animated mathematical conversations that continued uninterruptedly from the lecture hall into the cafeteria at one end of the corridor. It was hard to keep away from being a part of that excitement. I would go to every lecture and listen to some of the big players in the field like Ricardo Mañé, Jacob Palis and the entire school from IMPA. It was just after one of those brilliant lectures by Marcelo Viana that I turned around looking at others in the audience and saw an equally happy face greeting me with infectious enthusiasm: “Are you enjoying the workshop lectures too? Wasn’t it an amazing talk?” That’s how I first came to meet Stefano Luzzatto and we soon became great friends discussing the lectures.

All in all, it turned out that I stayed on for a year longer than my initial appointment of nine months. Towards the end of 1991 I decided to take formal Italian lessons running in town and also learnt about local traditions, experienced first hand typical Christmas and new year festivities of the region, the famous Carnival in February and thus ICTP and Trieste became an integral part of my inner world. I am still very nostalgic about that magical period of my life.
The charm of ICTP seemed to borrow from the alluring character of the town of Trieste itself, very aptly captured by the following lines of the poet Umberto Saba:
ha il cantuccio a me fatto, alla mia vita
pensosa e schiva.
In English translation:
has a nook made just for me and my life,
pensive and reserved.

With such a refreshing outcome coming to me at long last, I had found some stability of sorts in my mathematical adventures.

The town of Trieste situated right at the border of Italy has historically lived through varied emotions, poignantly captured by Jan Morris in her book titled Trieste and the meaning of nowhere, a compelling read that portrays the perspective of a different emotion. This book was a gift to me by another Italian teacher, Elisabetta Lippolis, a native of Trieste.
Even more recently, a couple of months back, on December 18, I came across the news in the daily Times of India that a translation into Italian of a celebrated Kannada novel was being released. The translator Jaya Murthy’s husband was the well-known mathematician late M.K. Venkatesha Murthy who earned his PhD from TIFR, Mumbai, and was later a long serving faculty member at the University of Pisa.
My mind pondered, and I wondered about these strange connections between mathematics, languages and cultural underpinnings that somehow stay alive and harmoniously blend themselves in adapted habitats. Even though I have only been an intermittent visitor to Trieste, the spirit of the town must be dwelling within me, and I was able to feel it palpably in my mother tongue through translation, so much so that I have indulged in reproducing it here. \blacksquare
Footnotes
- The colour branding for the sections of Centre has changed a bit since those early days, but luckily the Mathematics Section has sticked with the colour blue, though the format may have transformed over the years. ↩
- It appeared in the Journal of Molecular Evolution, vol. 33, (1991) pp. 105–113. ↩
- The Tata Institute of Fundamental Research (TIFR) in Mumbai is often referred to by many people as just `Tata’, for short. ↩
- It was published by AMS in their Mathematical Surveys and Monographs series, Volume 123. ↩
