Realising the hyperbolic: A parallel perspective

This expository article offers a unique take in exploring the beautiful landscape of hyperbolic geometry by ideating the construction of a “hyperbolic room” in a science museum.

The geometry that we have all learned in school is Euclidean geometry, based on a certain number of axioms that Euclid described in his great book The Elements. The fifth axiom is the “parallel” axiom: through a point outside of a straight line runs one, and only one, straight line that is parallel to the straight line.¹ Is it possible to imagine a geometry where all the axioms are valid except for the fifth one? This question kept a lot of mathematicians preoccupied for over 2,000 years and was answered in the beginning of the 19th century. Lobachevsky, Bolyai and Gauss proved that this is indeed possible: they described a coherent geometry without the parallel axiom, known as “hyperbolic geometry”.

This geometry was initially just an intellectual creation, not motivated by real application, but has gained more and more importance in mathematics, to the point where certain mathematicians now have a real intuition for it, as if they really lived “inside” this geometry. Hyperbolic geometry in three dimensions has been key to putting the famous Poincaré conjecture in a natural context, which led to the magnificent solution by Perelman of the geometrisation conjecture in 2006.

The question we will try to answer in this article is this: How could a science museum give its visitors a concrete idea of hyperbolic geometry? To try and answer that question, let us briefly explain some basic notions of a geometry where an infinity of parallel lines can be drawn through a point outside a given line.²

The world of Poincaré

To start building this world, we must first leave our “Euclidean world”, the one we learn about in school, and move to a hyperbolic world. There are different models to represent hyperbolic geometry in the Euclidean setting. We will only look at what is called the Poincaré disc model. The Poincaré disc, one of the models of hyperbolic geometry, is a world that occupies a disc of radius 1 (if one observes it with Euclidean eyes). If an inhabitant of this world leaves from the centre of the disc towards the edge and if the journey is made at constant speed, it will take him an infinite time to reach the edge of the disc, which means that he will never reach it! The distance, called “hyperbolic distance”, from the centre to the edge is infinitely long. This means that points outside the disc, which we can see because we live in the Euclidean world, do not belong to the universe of the hyperbolic plane, and the inhabitants of the hyperbolic plane do not perceive these points. In this model, “(hyperbolic) straight lines” are arcs of circles that are orthogonal to the boundary unit circle.

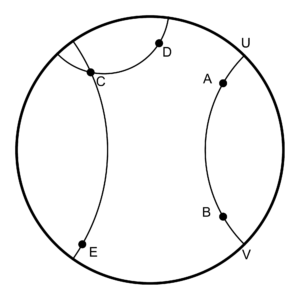
Figure 1 shows the “hyperbolic straight lines” connecting the points A and B, C and D, and finally C and E. The Euclidean circle arcs CD and CE do not intersect the arc AB, which means that CD and CE are parallel to AB. Two straight lines are parallel if they do not intersect (or if they coincide). In Euclidean geometry, two straight lines that are parallel to a third line are parallel to each other. This is clearly not the case in hyperbolic geometry, and so our intuition is not a good guide here. What we are also used to, is that two parallel lines are everywhere at the same distance from each other, but in hyperbolic geometry, this is not true either. The hyperbolic distance \bar{d} between points A and B can be defined by \bar{d} = \frac{1}{2}\vert ln (\frac{AV\cdot BU}{AU\cdot BV})\vert, where UV is a Euclidean circular (Figure 1) arc that is orthogonal to the boundary circle and connects A and B and AV, BU, AU, BV are the (Euclidean!) distances between the points. If either A or B approach U or V then this distance becomes infinitely large: the rim of the disc is not part of the Poincaré disc, it is called the horizon “at infinity”.

Euclidean and non-Euclidean tilings

In order to give the museum visitor the illusion that he finds himself in a hyperbolic world, we would need to put him on a disc so that the rim is invisible as it is infinitely far away. We would also need to give visitors the illusion that light beams follow paths that are circle arcs instead of straight lines. All this is not easy! Let us first look at some other properties of hyperbolic geometry.

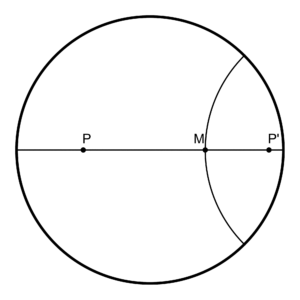
In Figure 2, points P and P' are symmetrical about the hyperbolic straight line passing through the point M and perpendicular to PP'. The hyperbolic distances \overline{MP} and \overline{MP'} are identical. We should bear in mind that we are referring to hyperbolic symmetry, and not the Euclidean one!

One can verify, using the hyperbolic distance formula, that the point P' is obtained by inversion³ along the circle representing the hyperbolic straight line passing through M and perpendicular to PP'. If C is the centre of this circle, we have CP' = \frac{R^2}{CP}, where R is the radius of the circle (Euclidean distance from C to M).

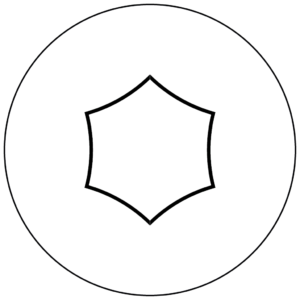
Let’s apply this notion of symmetry to tilings. If we want to pave the Euclidean plane with regular polygons, we have a choice between triangles, squares and hexagons. With triangles, six of them will meet at each vertex (Figure 3a). With squares, the number is four (Figure 3b), and with hexagons, it is three, as in Figure 3c.

In our hyperbolic plane, the situation is quite different. To start with, the sum of the angles of a triangle is not equal to \pi anymore, in fact, the sum is always smaller than \pi.

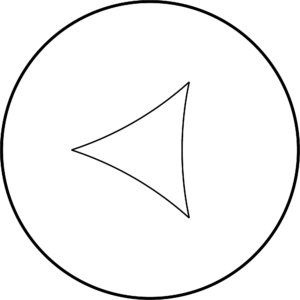
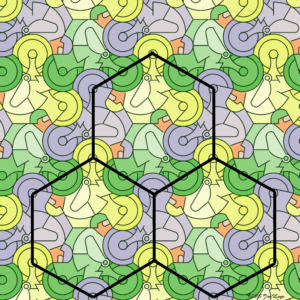
Figure 4 shows an equilateral triangle where the sum of the angles is 3\pi/4 (the angle between two curves is measured by measuring the angle between the tangents to the two curves, at the point where they intersect). Figure 5 shows an equilateral triangle where the sum of the angles is equal to zero as both the circular arcs at any vertex are orthogonal to the boundary circle on which the vertex lies.

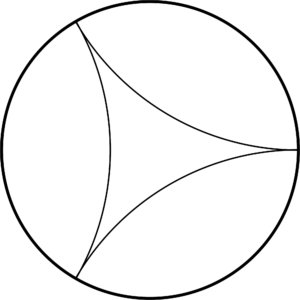
This means that one can draw equilateral triangles with any angles between 0 and \pi/3, and that one can draw an infinity of tessellations by equilateral triangles.The same thing is true for the other regular polygons. How can we draw a tiling by regular (hyperbolic!) polygons with n vertices so that p polygons meet at each vertex?

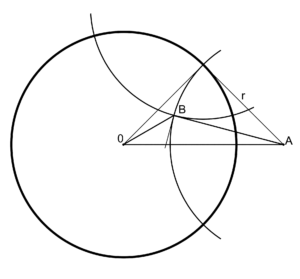
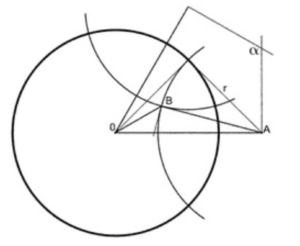
In Figure 6, we have to find the position of the centre A and the radius r of a circle so that:

• The circle is orthogonal to the unit circle.
• The angle between this circle, and a second circle with the same radius, rotated by an angle 2\pi/n around the origin, equals 2\pi/p.

In the triangle ABO, the angle \widehat{BOA} equals \pi/n, and the angle \widehat{OBA} equals \pi/p + \pi/2. As AO = \sqrt{1 + r^2} and AB = r, we can easily find r.
This construction is only possible if \pi/n + \pi/p + \pi/2 < \pi, from which follows the general rule for hyperbolic tilings:

\frac{1}{n}+\frac{1}{p} < \frac{1}{2}.

In order to draw the polygon, we rotate the point A around the origin by an angle 2\pi/n, and we draw the circular arcs of radius r. If we take n = 6 and p = 4, this gives us the hexagon in Figure 7.

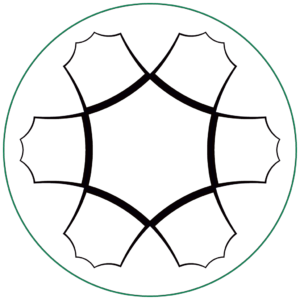
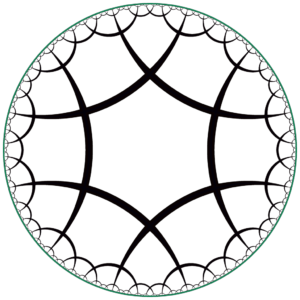
So this is a fundamental tile. As p = 4, the angles of the polygon are all \pi/2. In order to find the neighbouring tiles, all we need to do is to draw the symmetric tiles in a hyperbolic way. The axes of symmetry are the sides of the polygon. As we have seen, symmetry in the hyperbolic plane means inversion in a circle. The fact that the circle inversion preserves angles, ensures that the images by inversion (in the circles that make up the sides of the polygons) will also have angles of \pi/2. We then obtain the picture in Figure 8.

We can repeat this as many times as we want (see Figure 9).

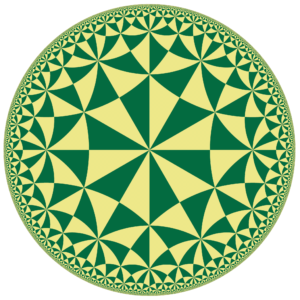
This is the tiling that M.C. Escher used in his famous “Cirkellimiet 4, Angels and Demons” (Figure 10). Note that Escher carved this on wood by hand!

M.C. Escher took this tiling, but he obviously did not use the images of the tiles obtained by inversion. At each inversion, the image was turned by 180 degrees, which assures that the demons and angels match up perfectly. Figure 11 gives two other examples. On the left is the same (6,4) tiling, this time with triangles in alternating colours, and on the right is a (3,18) tiling.

Back to the science museum.

We now know how to tile the hyperbolic plane with tiles that are obtained by circle inversions. How does this help us in our construction of the museum?

Remember those “Galleries of Mirrors” with all sorts of mirrors that deform the reflection of the spectator into bizarre shapes? In order to lose some weight, all one needed to do was to stand in front of a cylindrical mirror with a vertical axis (Figure 12).

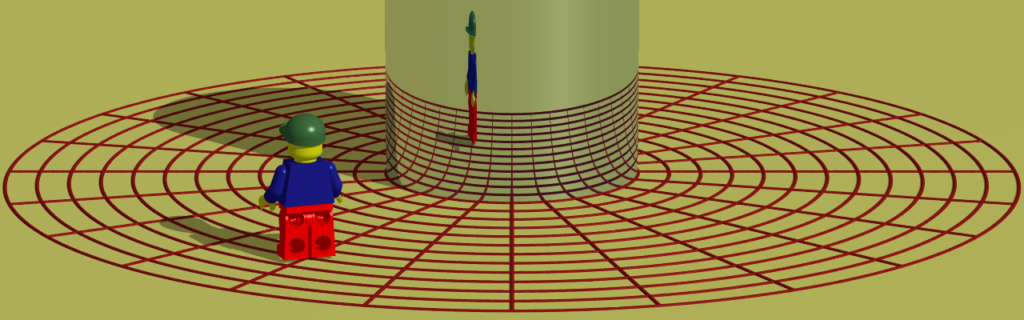
So here is our idea for the museum: We build a chamber where the walls are cylindrical mirrors. We choose the radii and positions of the mirrors as if we were constructing the base tile of a hyperbolic tiling.

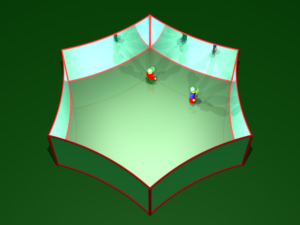
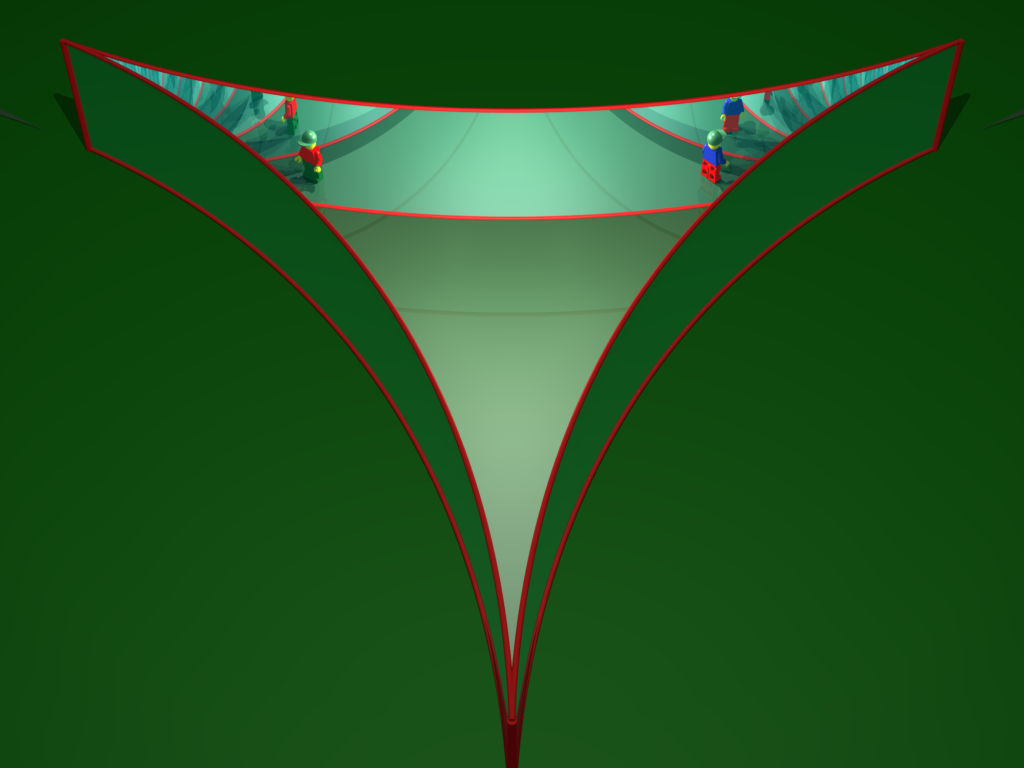
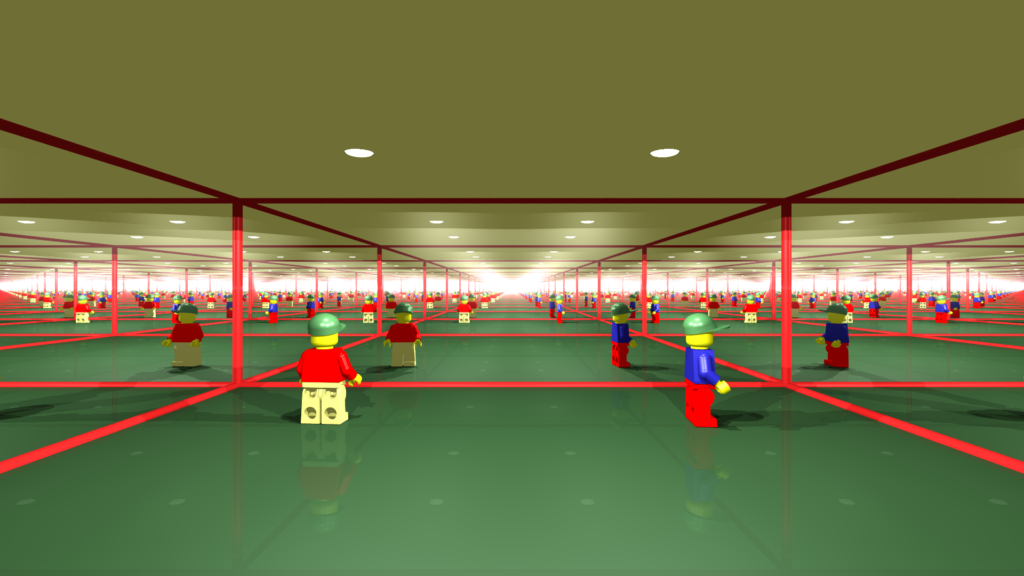
Figure 13 depicts what such a chamber might look like. (At the moment, there are just two visitors, and we still have to construct a way in and out!)

Will the visitor’s view in this chamber be exactly like the view in a real hyperbolic world? Unfortunately, no! Let’s not forget that straight lines in the hyperbolic world, i.e., lines that light beams will follow, are Euclidean circle arcs. This means, for instance, that a visitor in one corner of the room should be able to see all the other corners, but this is not the case here! The chamber itself is not hyperbolic.

Nevertheless, it can be shown that the view inside the chamber and all the reflections on the walls do not differ much from the view in a real chamber of the hyperbolic world. The mathematical explanation for this is outside the scope of this article.

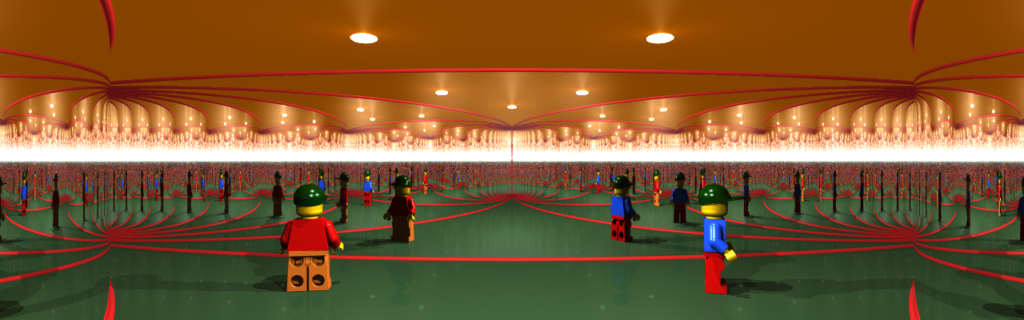
Let’s put our camera inside the chamber.

The camera captures a picture like in Figure 14. With good quality mirrors, the visitors will experience the illusion that they find themselves on an infinitely large plane, just like the inhabitants of the hyperbolic world, who cannot see the edge of the disc either.

For the shape of the chamber, the possibilities are endless. Figure 15 shows a chamber according to the (3,100) tiling,and the view on the inside is shown in Figure 16.

For comparison, Figure 17 shows a purely Euclidean chamber.

How can we make the experience even more spectacular for the visitors in the museum?

So far we only talked about the hyperbolic plane, but we can also talk about hyperbolic space. Instead of the Poincaré disc, let’s take the Poincaré ball, the interior of a unit ball in Euclidean 3-space. In this ball, “hyperbolic planes” are pieces of spheres that are orthogonal to the unit sphere. Symmetries can now be described by inversions in these spheres.

This hyperbolic space can also be “tiled” by regular polyhedra. In our Euclidean world, the only regular polyhedron capable of such a tiling is the cube, but in the hyperbolic world, we do have choices! We will see that the choices are not as abundant as in the hyperbolic plane. Note that the hyperbolic space that we are referring to is three-dimensional. One can also discuss higher-dimensional hyperbolic spaces, and the possibilities to tile that space, but this would lead us too far . Suffice it to say that it has been shown relatively recently that such a tiling is impossible in dimensions higher than 30, a theorem dating from 1981 by the Russian mathematician Èrnest Vinberg.⁴

Hyperbolic regular polyhedra are objects with faces that are pieces of spheres. Look at Figure 18: this can be considered as a cut through the Poincaré ball by a plane passing through the origin.The angle \widehat{BOA} is now equal to (\pi - \alpha)/2, with \alpha the dihedral angle of the Euclidean polyhedron.⁵ In order to be able to construct tilings where p polyhedra meet at each edge, the condition is:

\frac{\pi}{p} < \frac{\alpha}{2}. There is also a second condition: the spheres that make up the faces of the polyhedron must be orthogonal to the boundary unit sphere, which creates a maximum for p: for polyhedra where three faces meet at a vertex, the maximum value of p is 6, for 4 faces this is 4, and for 5 faces it is 3.333…. All this limits the possibilities for tilings by hyperbolic regular polyhedra to the following:

• Tetrahedron with dihedral angles of 60^{\circ} with “vertices” on the unit sphere (a purist opinion could be that this is not really a polyhedron as the vertices are at infinity, and the inhabitants of the hyperbolic ball cannot see them!).
• Cube with dihedral angles 72^{\circ} or 60^{\circ}. In the latter case, the “vertices” are at infinity.
• Octahedron with dihedral angles 90^{\circ}.
• Dodecahedron with dihedral angles 90^{\circ}, 72^{\circ} or 60^{\circ} (in the latter case the “vertices” are at infinity).
• Icosahedron with dihedral angles 120^{\circ}.

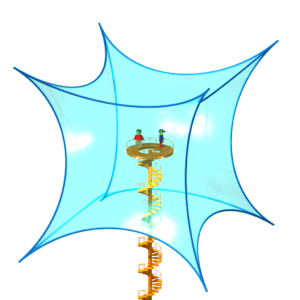
For the idea of the museum, we could consider a chamber with spherical walls, arranged to obtain a tiling of hyperbolic space.

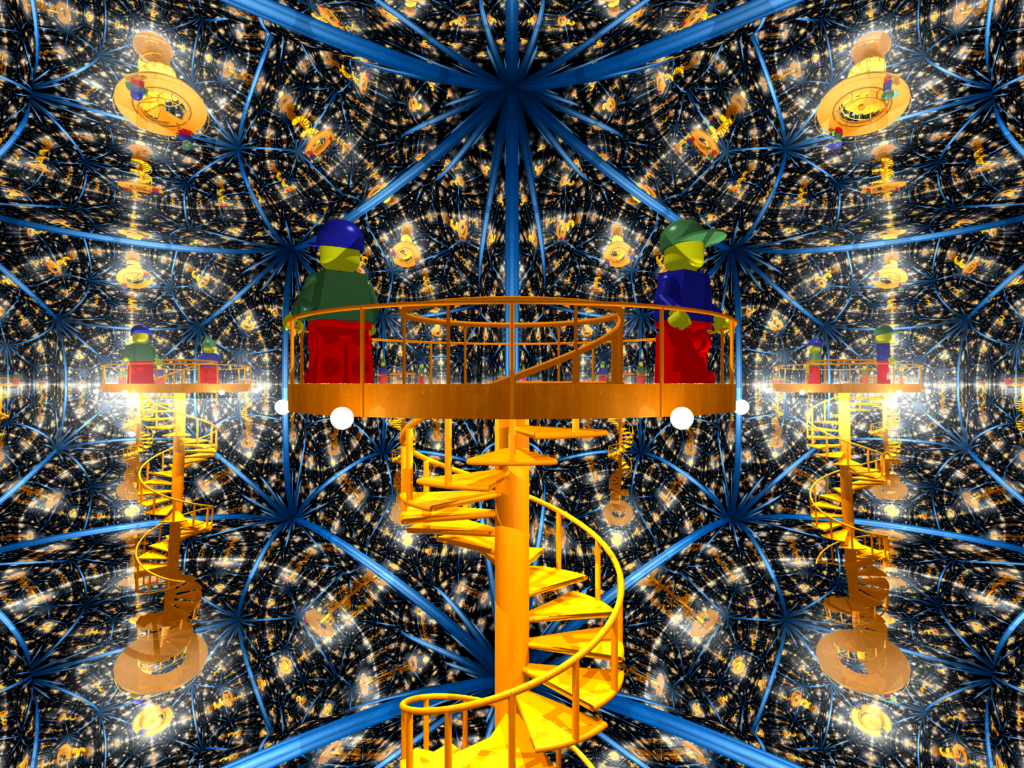
Figure 19 shows a chamber in the shape of a hyperbolic cube. Figure 20 depicts a view inside the chamber.

If you prefer a chamber in the shape of a dodecahedron, see Figure 21. Figure 22 shows the view inside the chamber.

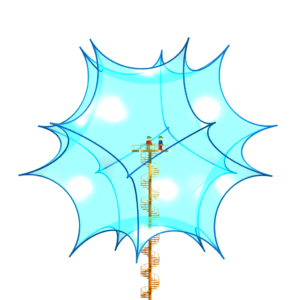And finally, Figure 23a shows an icosahedral chamber, with Figure 23b showing the view inside.

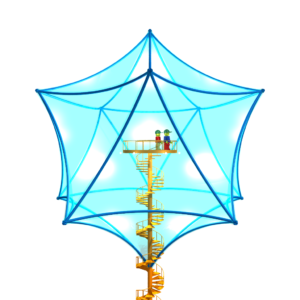Annexe by Étienne Ghys

Why is the view inside these rooms close to that which a hyperbolic inhabitant would have?

What an observer sees is of course transmitted by light rays which penetrate his eye. In the museum room, rays follow rectilinear paths and are reflected on mirrors according to the well-known law of light reflection: the angle of incidence is equal to the angle of reflection. For a hyperbolic inhabitant, in his hyperbolic room, it is the same thing, except that his light rays follow hyperbolic lines, which, as we have seen, are arcs of circles for a Euclidean observer (us!). A priori therefore, there is no connection between the two visual experiences!

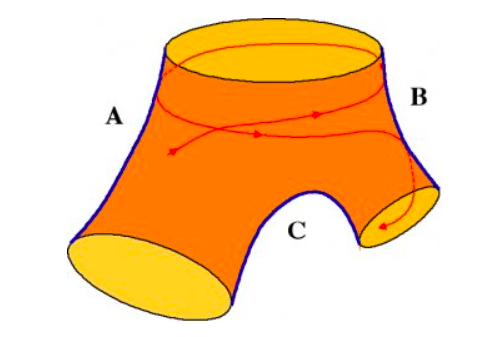
Hyperbolic geometry is the realm of negative curvature. This is an area of research that has exploded in the past 30 years, particularly due to the fact that it is an extremely powerful method for studying fundamental questions of topology. Initially, it concerned the study of surfaces which locally resemble a horse saddle, such as, for example, in Figure 24.

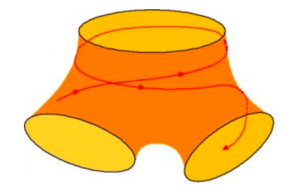
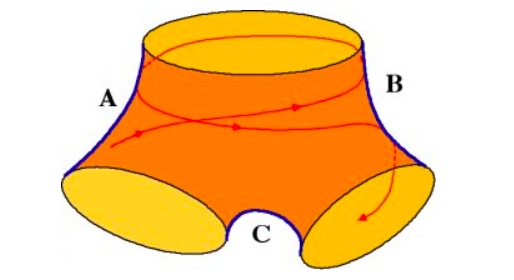
Jacques Hadamard, at the beginning of the 20th century, tried to understand the behaviour of what are called geodesics on such a surface: they are curves drawn on the surface which, locally, are the shortest from one point to the other (for example, the red curve in Figure 24). “Mechanically”, they are the trajectories of a point object which moves on the surface without any friction and is not subjected to any force, except for the reaction which forces it to remain on the surface. Contrary to all expectations, he found that the behaviour of these geodesics is insensitive to the surface and depends only on its topology. For example, if we consider the two surfaces in Figures 25 and 26, we can associate with any geodesic of one a geodesic of the other which has precisely the same qualitative behaviour: two associated geodesics travel on surfaces in the same way in the sense that, for example, they cut the “seams” marked A, B, C in the two figures in the same order.

During the 1980s, due mainly to the impetus of Mikhail Gromov, objects with negative curvature left the world of smooth objects like surfaces, and a large number of singular objects, like graphs, for example, qualified as hyperbolic, and we now assign them a curvature .

Let’s go back to our rooms! The hyperbolic observer who wants to understand the motion of light rays in his room can do the following. Let’s take two copies of the fundamental triangle and glue them along their sides. We get a somewhat bizarre object that mathematicians call an “orbifold”. This orbifold is negatively curved in the generalized sense to which we were referring. The geodesics on this object walk on one of the two faces by tracing a usual hyperbolic geodesic and when they arrive on a side, on which the other face has been glued, we can think that the geodesic continues on its path on the other face and the property that the angle of incidence is equal to the angle of reflection is nothing other than the assertion that if we unfold our object along the seam, the trajectory is smooth and does not snap! In plain English, our hyperbolic observer “sees” the geodesics of an orbifold with negative curvature! Perhaps this is a way of saying simple things in a complicated way!

What does the museum visitor do? The same thing: except that he glues two Euclidean triangles with circular sides along their sides. The object he obtains is still an orbifold. Is it negatively curved? No, because inside the chamber, the geometry is so Euclidean that the curvature is zero…. But it turns out that the curvature is still negative or zero…. It is strictly negative along the seams because of the concavity of the edges! Gromov was able to generalise Hadamard’s theory to all these bizarre objects and it turns out that the two orbifolds, that of the Euclidean visitor to the museum and that of the hyperbolic being, even if they are not the same, have the same topology and, as Hadamard had shown, to each geodesic of one, that is to say to each light ray of one, we can associate a light ray of the other. Two associated light rays have the same behaviour in the sense that they are reflected on the walls in the same order! Concretely, this means that unless the visitor is an experienced physicist who enters with his measuring instruments, he will think that he is inside a hyperbolic chamber!\blacksquare

Footnotes

1. This is an equivalent version of Euclid’s original formulation . ↩
2. Another example of a non-Euclidean geometry is the geometry on the sphere: here, not a single parallel can be drawn through a point outside a line. The `lines’ on the sphere are the great circles, and two great circles always intersect each other. This geometry is called “elliptic” . ↩
3. Inversion along a circle is a transformation that sends points inside the circle bijectively to points outside it and vice-versa. In the hyperbolic world, since hyperbolic straight lines are circular arcs, to invert along such arcs means to reflect along straight lines. In particular, inversions preserve angles and distances between points . ↩
4. “The nonexistence of crystallographic reflection groups in Lobachevskiĭ spaces of large dimension.” (Russian), Funktsional. Anal. i Prilozhen. 15 (1981), no. 2, 67–68 . ↩
5. A dihedral angle is the angle between two intersecting planes. Here, it is the angle between two intersecting faces at a vertex of the polyhedron . ↩