Mathematics in India

Validity of Certain Mathematical Expressions in Calculus and Algebra

Before discussing the validity (or otherwise) of division by zero, we present a couple of examples to illustrate that a mathematical expression which may not be valid in the framework of basic calculus may well be valid in the framework of sophisticated algebra!

Question (i). Is the following expression valid?

\[\begin{equation}\label{GP}
1+2+2^2+\cdots = -1
\end{equation}\]

A scholar in mathematics, whose thinking goes in the direction of an infinite G.P. series of usual numbers, will exclaim:

Answer 1 (Calculus viewpoint). No, it is absurd!

And, is likely to conclude that a person with low mathematical maturity has blindly applied the formula for the sum of an infinite G.P. series without considering the convergence of the series, i.e., the restriction on the common ratio.

However, a reader steeped in algebra or algebraic number theory will recognize relation (\ref{GP}) to be a valid expression!

Answer 2 (Algebra viewpoint). Yes, (\ref{GP}) holds in the `2-adic completion’ of the ring of integers \mathbb Z (=\{0, \pm 1, \pm 2, …, \}).

For readers unfamiliar with the concept, we briefly describe the `2-adic completion’ of \mathbb Z.<sup>1</sup> or \pi on it missing; one `completes’ this to the real number line by including all the irrational numbers. The main tool here is the absolute value of a number, which gives its distance from the origin, and helps define a metric on the real line as the absolute value of the difference between any two numbers.

Any nonzero integer r can be uniquely expressed as 2^mq for some m \ge 0 and odd integer q. We define the 2-adic norm of such a nonzero integer r to be

||r||_2:=2^{-m} and define the norm of 0 to be

||0||_2:=0.It can be verified that, for any x,y \in \mathbb Z,

d(x,y):= ||x-y||_2defines a `metric’ or a distance function on \mathbb Z, making ({\mathbb Z}, d) a metric space. Under this metric, two numbers x,y are envisaged to be `close’ if their difference x-y is divisible by a high power of 2. The 2-adic completion of \mathbb Z is the completion of \mathbb Z with respect to this metric. It is an extension of \mathbb Z, and is denoted by {\mathbb Z}_2.²

Recall that any positive integer r has a unique 2-adic expansion of the form

r=2^m(a_0+a_12+a_2 2^2+ \cdots +a_n2^n),where m \ge 0 and each a_i is either 0 or 1 with a_0 =1.

It can be shown that any element r in {\mathbb Z}_2 can be represented in the form of a (possibly infinite) series

r=2^m(a_0+a_12+a_2 2^2+ \cdots ),where m \ge 0 and each a_i is either 0 or 1.

One can now see that the relation (\ref{GP}) is a precise relation in the ring {\mathbb Z}_2. Such rings are now of central importance in algebra and number theory.

Question (ii). Is the following expression valid?

\[\begin{equation}\label{GP2}
1+x+x^2+ \cdots +x^n+ \cdots=\frac{1}{1-x}
\end{equation}\]

Again, if one thinks in terms of calculus, as an infinite G.P. series, one would give a conditional answer:

Now recall ([5], p. 45) that over the field of real numbers {\mathbb R}, expressions of the form

\[\begin{equation}\label{powerseries}
a_0+ a_1x+a_2x^2+ \cdots ; a_i \in {\mathbb R} {\rm \ for \ all \ }i,
\end{equation}\]

with possibly infinite terms, are called power series over {\mathbb R}. (Late S.S. Abhyankar would humorously refer to a power series as a polynomial with the last term deleted.) One can add, subtract and multiply two power series over {\mathbb R} to get another power series over {\mathbb R}. Thus the collection of all such power series over {\mathbb R} forms a ring, called the power series ring, denoted by {\mathbb R}[[x]]. This structure is of paramount importance in commutative algebra and algebraic geometry.

(1-x)(1+x+x^2+ \cdots +x^n+ \cdots)=1and hence (\ref{GP2}) holds.

Thus, if one interprets (\ref{GP2}) as a statement in the power series ring {\mathbb R}[[x]], one would regard it as a valid statement, unconditionally. Thus:

Answer 2 (Algebra viewpoint). Yes, it is a valid identity in the power series ring {\mathbb R}[[x]].

This brings us to the issue of the mathematical validity of expressions of the form (\ref{powerseries}). About three centuries before the power series ring {\mathbb R}[[x]] was constructed rigorously, Isaac Newton freely used power series of the form (\ref{powerseries}) and we now recognize, in retrospect, that, mathematically speaking, he was considering elements of the ring {\mathbb R}[[x]] (without perhaps being conscious of such an abstract structure). He was thus dealing with valid mathematical expressions.

We now know that Newton’s work is valid when viewed in the framework of the structure {\mathbb R}[[x]] and that, in such a ring, it is rather expressions of the form -a \le x \le b or -a < x < b which do not make sense.

The above history has been summed up by Abhyankar in his inimitable style in his celebrated article (1976) “Historical Ramblings in Algebraic Geometry”³(see [2], p. 417):

The purpose of discussing the above examples is to remind ourselves that it is not desirable for us to arrive at hasty conclusions about the validity of any concept or construct introduced by mathematicians belonging to a different era on the basis of our present-day conventions and notation. Leaving aside the question of fairness, we may possibly be losing out on fresh mathematical insights dormant in the work on those concepts or constructs.

We thus need to approach the theme of `division by zero’ in ancient Indian algebra with an open and receptive mind.

Division by Zero: Undefined or Impossible?

Division by zero is declared to be `undefined’ in current mathematics. That the concept is treated as undefined has led to a deep-rooted conviction, even among professional mathematicians, that `division by zero’ or a `fraction with zero-denominator’ is `impossible’, `meaningless, `absurd’, etc.⁴

However, as we shall see, Brahmagupta declares 0 \div 0=0 and for a \not =0, both Brahmagupta and Bhāskarācārya define a \div 0 (or \frac{a}{0}) to be a new number which we denote by \infty.

Admiring modern writers sometimes tend to gloss over statements by ancient Indian mathematicians on division by zero; the more objective authors frankly call them errors in otherwise remarkable texts; a few charitable authors gently remind us that our mature understanding of the issue evolved over a long period of time, a benefit the ancient greats did not have. Needless to say, the statements on division by zero provide welcome ammunition to authors on the look-out for opportunities to debunk ancient Indian stalwarts.

the algebraist was freed from the shackles of analysis, or rather (as in Vedanta philosophy) he was told that he always was free but had only forgotten it temporarily

There is a standard argument, going back to the German mathematician Martin Ohm (1828), which tries to establish the impossibility of division by zero: “If a is not zero, but b is zero, then the quotient a/b has no meaning” for the quotient “multiplied by zero gives only zero and not a, as long as a is not zero.” (Quoted in [4], p. 246)

Now suppose a is a non-zero integer. The problem with the above argument is that it assumes that cross-multiplication extends to numbers of the form \frac{a}{0}, i.e.,

\frac{a}{0}=x \Rightarrow a = 0 \times xand that the rule 0 \times x= 0 holds even for numbers x of the form \frac{a}{0}.

What would be the problem in considering numbers of the form \frac{a}{0} in a mathematical system where cross-multiplication is not allowed in the above case or where 0 \times x is considered undefined when x is a number of the form \frac{a}{0}?

Various modern authors try to give the following version of the above argument to show the `impossibility’ of division by zero (we shall quote a few such passages later):

Suppose m, n are distinct integers. If \frac{m}{0}=\infty =\frac{n}{0}, then `cross-multiplication’ gives m=0 \times \infty = n, a `contradiction’.

This argument by contradiction overlooks two questions.

Question 1. What if \frac{m}{0} and \frac{n}{0} are considered unequal when m \ne n?

Question 2. What if cross-multiplication is not permitted for such zero-divided numbers, i.e., for numbers with zero in the denominators?

Question 2 above leads us to wonder: can one not have a valid number system with (possibly new) numbers y=\frac{x}{0} with suitable restrictions on the operation 0 \times y (for instance, by treating 0 \times \infty as undefined) or with restrictions on the validity of cross-multiplication? That is, can we not permit (or introduce) new numbers y=\frac{x}{0} but without the implication \frac{x}{0}=y \Rightarrow x=0 \times y?

After all, using such an argument by contradiction, we may also claim that one cannot multiply by zero; since m \times 0=0= n \times 0 would imply, by cancelling zero, that m=n. We avert this, by making the rule that the `cancellation principle’ m \times x= n \times x \Rightarrow m=n will not be applied in the case x = 0. What prevents us from making such a restriction for cross-multiplication too?

Indeed, setting \infty :=\frac{1}{0}, the relation \frac{m}{0}=\frac{n}{0} takes the form m \infty=n \infty and if we make the rule that the cancellation principle m \times x= n \times x \Rightarrow m=n will not be applied in the case x = \infty (just as it is forbidden in the case x =0), then there is no contradiction!

We may recall an analogous situation from the past: there was a time when square roots of negative numbers were considered non-existent and forbidden; even the validity of subtracting a bigger number from a smaller number (i.e., the existence of negative numbers) took a long time to gain universal acceptance. An argument by contradiction had been used to show that \sqrt{-1} does not exist. It was said that the rule

\sqrt{a}\sqrt{b}=\sqrt{ab}would yield

-1= \sqrt{-1}\sqrt{-1}=\sqrt{1}=1,a contradiction.

But now \sqrt{-1} is accepted by putting suitable restrictions on the use of the rule \sqrt{a}\sqrt{b}=\sqrt{ab}. Can we not then conceive of fractions \frac{x}{0} with suitable restrictions? Indeed, in some areas of modern mathematics, versions of \frac{x}{0} do get adopted through suitable euphemisms or formalisms.

Enterprising modern mathematicians have created elaborate legitimate (or technical) machinery to overcome the limitations imposed by the prohibition against use of zero in the denominator. The most familiar are the methods of calculus with its concept of limit, results like l’H\hat{{\rm o}}pital’s rules, and a language which enables one to express intuitive ideas like \frac{1}{0}=\infty through permitted euphemisms. Less well-known are the devices of commutative algebra, algebraic geometry and algebraic number theory like `localization’ which describes a legitimate structure for directly writing fractions with zero in the denominator without any subterfuge, and the more sophisticated ideas of `valuation theory’ which admit multiple levels of infinities and thereby provide higher-dimensional algebraic analogues of l’H\hat{{\rm o}}pital’s rules. Abstract measure theory too provides us with examples.

We shall now quote rules in modern mathematics in the above areas which are really the counterparts of the rules on division by zero or zero-divided numbers in ancient Indian mathematics.

These rules demonstrate that our decision to declare `division by zero’ as `undefined’ is only a convention. It is a safe and convenient convention, but still a convention. The convention is made to nip in the bud some potential difficulties which would require careful handling. We do not usually dare to conceive of any mathematical framework with a different convention permitting fractions with zero in the denominator.

We shall show that while we have confined ourselves to certain safe conventions regarding the zero, there could be other approaches where the ideas of Brahmagupta and Bhāskarācārya on zero-divided numbers, including certain apparently erroneous examples of Bhāskarācārya, will appear not only valid but even natural.

It is to be hoped that these mathematical considerations will make future authors refrain from saying that the ancients who did not follow our present convention on division by zero, committed errors.

Commutative Algebra: the Ring of Fractions

For any multiplicatively closed subset S of a commutative ring R, one constructs a new ring S^{-1}R, where the elements of S⁵ become invertible by introducing fractions of the form a/b, where a \in R and b \in S. Two fractions are defined to be equal if they satisfy a certain equivalence condition.⁶ Now, S is allowed to include zero!

If 0 \in S, then all elements in the extended ring become the zero element of S^{-1}R=\{0\}. In particular, we have 0/0=0; in fact, in this construction, a/0= 0 even when a is a non-zero element of R.

This is clearly an instance of rigorous formal modern mathematics which permits fractions with zero in denominator and accommodates expressions like 0/0=0.

When 0 \in S, the ring S^{-1}R does not have a rich structure; it is literally a trivial ring. In the next section, we show that in the modern theory of abstract integration, a close analogue of Brahmagupta’s rule 0/0=0 is used in a more substantial way.

Setting \infty:= \frac{1}{0} (an identification actually made by Brahmagupta in an astronomy chapter Brāhma-Sphuṭa-Siddhānta, VII v 14), the rule 0/0=0 may be envisaged as 0\cdot\infty = 0. This is precisely the modern convention adopted in measure theory and probability theory.

A Convention in Measure Theory

Consider the following excerpt from Real and Complex Analysis by W. Rudin ([19], pp. 19–20):

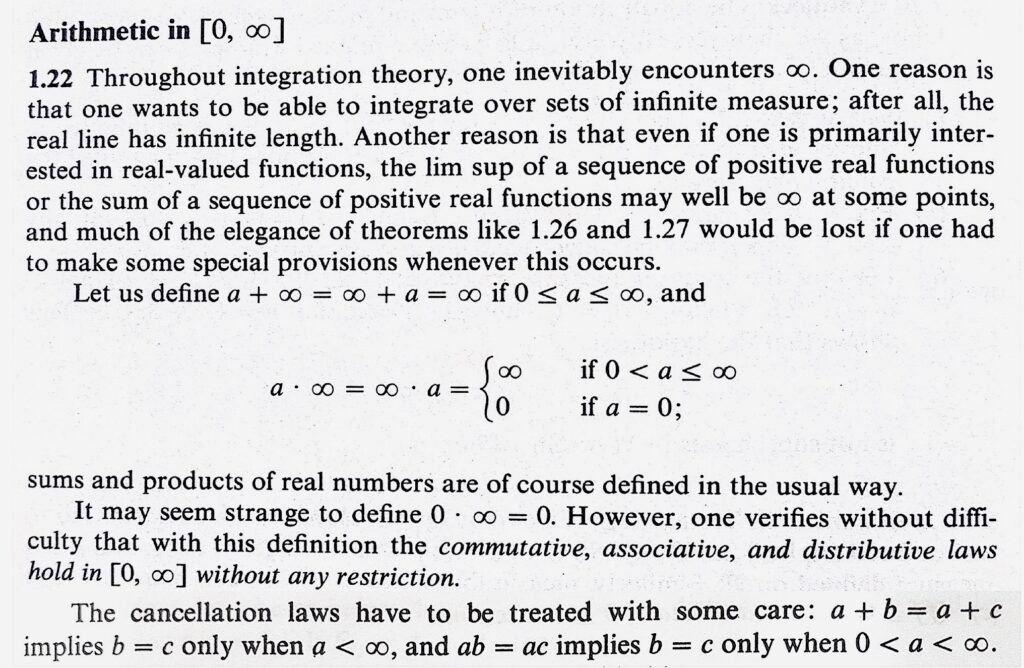
We again emphasize that the definition in Rudin’s book 0\cdot\infty = 0 corresponds to the much criticized Brahmagupta statement 0/0=0 and there is no logic in accepting the former and not the latter.

The last rule

ab=ac \ \text{ implies } \ b=c \text{ \ only when \ } 0< a <\infty, i.e., the cancellation law is not to be applied when a=0 or a=\infty is the issue we discussed before. In the context of the Indian works on zero-divided numbers, the above restriction on a=\infty corresponds to saying that from the relation \frac{m}{0}=\frac{n}{0}, one is not to deduce m=n. As we had discussed earlier, the argument by contradiction used to invalidate zero-divided numbers collapses. Again, there is no logic in accepting the modern convention described in Rudin and condemning the corresponding convention in ancient Indian texts.

A Convention in Valuation Theory

We now give another illustration of a convention from valuation theory in commutative algebra, algebraic geometry and algebraic number theory, which has a striking resemblance with ancient Indian conventions. We shall quote a passage from the book Commutative Algebra by N. Bourbaki, a collective pseudonym of a group of strong mathematicians whose textbooks on various advanced branches of pure mathematics are considered epitomes of rigorous presentation. The passage occurs in the chapter on Valuations (Chapter VI), in the section on Places (Section 2).

Let K be a field and let \tilde{K}:= K \cup \{\infty\}. Bourbaki writes ([3], pp. 381–382):

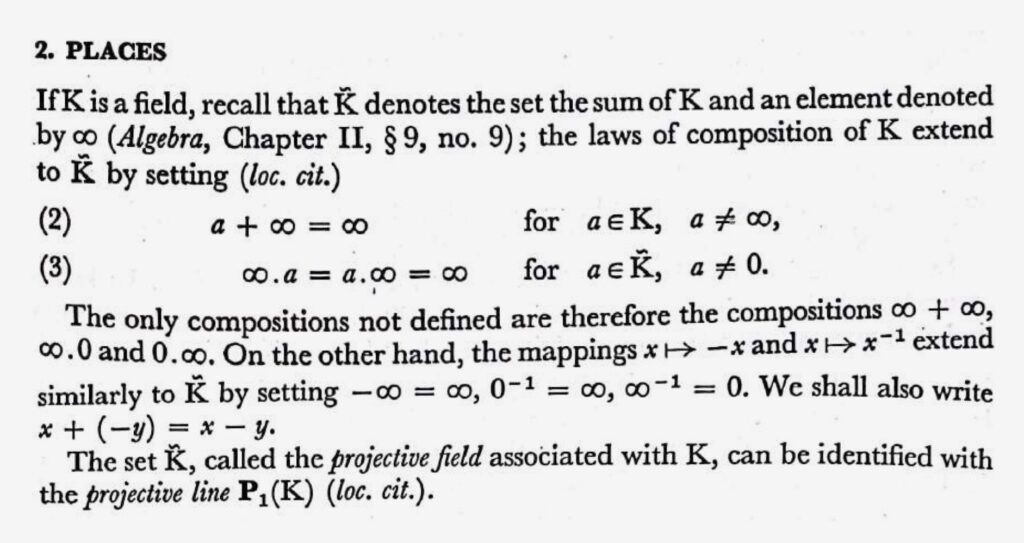
Note that conventions are not unique in modern mathematics and vary from context to context. We have just now seen that 0 \times \infty=0 in abstract measure theory but undefined in valuation theory.

As per the convention described in Bourbaki’s book quoted above, when K is the field of real numbers \mathbb R, a point \infty is added to \mathbb R to obtain the extension \tilde{\mathbb R}, and the following rules (among others) are made over the extended set: \frac{1}{0}=\infty, \frac{1}{\infty}=0 and a + \infty = \infty for all a \in \mathbb R. These are precisely some of the rules which appear in the Brahmagupta-Bhāskarācārya tradition and are declared errors in modern articles and books on the history of mathematics.

Postponement of Evaluation

For understanding certain concepts and steps in the mathematics of zero in ancient Indian texts, we briefly discuss the technique of `postponement of evaluation’.

Let us consider the computation of \underset{x \to 0}{\lim} \frac{2x^2+x^3}{x^2+x^3}.

Let us leave aside for the time being the formal definition of limit in the Cauchy-Weierstrass (\epsilon - \delta) tradition and try to write down the steps actually taken (perhaps privately and not officially) by almost anyone who computes the limit for practical purposes.

When one sees that a simple evaluation by substituting x=0 in the given expression leads to the problematic \frac{0}{0} form, one sort of postpones the evaluation at x=0. Instead, one treats x to be a non-zero number and simplifies the rational expression to arrive at

\frac{2x^2+x^3}{x^2+x^3}=\frac{x^2(2+x)}{x^2(1+x)}=\frac{2+x}{1+x}Now, only at the end, one substitutes x=0 in the simplified expression \frac{2+x}{1+x}.

One of the most useful areas of investigations in number theory involves calculating expressions `modulo p‘ for a prime number p. The `postponement of evaluation’ is a standard practice in modern algebra and number theory when evaluating a rational expression modulo p. If one evaluates the numerator and the denominator separately modulo p, it could lead to a problematic \frac{0}{0} type expression.

Indeed, one should first suspend the evaluation p\equiv0, simplify the rational expression, treating all numbers as usual integers, and, only at the final stage, evaluate the given rational expression. We explain with an example.

Example. Compute the binomial coefficient \binom{21}{3} modulo 3.

Here, both 21 and 3 are zero modulo 3. While computing

\binom{21}{3}=\frac{21 \times 20 \times 19}{3 \times 2 \times 1}modulo 3, the identifications 21 \equiv 0 and 3 \equiv 0 are not to be made in the numerator and the denominator at the outset. Instead, the expression for \binom{21}{3} is first simplified to obtain

\binom{21}{3}=\frac{21 \times 20 \times 19}{3 \times 2 \times 1}=7 \times 10 \times 19.Now one can evaluate the RHS modulo 3. Since 7 \equiv 1, 10 \equiv 1 and 19 \equiv 1 modulo 3, we obtain \binom{21}{3} \equiv 1 modulo 3.

Here, there has been no limit calculation (the question does not arise); only algebraic substitutions. Although we are interested in numbers modulo 3, we postpone the use of 3 \equiv 0 till the penultimate step.

Ancient Indian algebraists anticipate this idea of postponement of evaluation in their work related to division by zero.

Some Remarks in Popular Books and Articles

Once a Ph.D. student of ISI had tried to point out an `error’ to the eminent statistician late Jayanta Kumar Ghosh; there was actually no error. The student perceived an error due to his misunderstanding. Ghosh gently told the student that errors can happen but when one is to point out the error of someone else, one has to be doubly careful. The present author was a witness to the incident when he was a master’s student at ISI more than 35 years ago.

When it comes to commenting on the works of the ancient greats of Indian mathematics, it is often found that authors do not follow such basic caveats that Ghosh referred to. In some of the passages mentioned below, the authors not only try to denigrate the novel approaches taken by the Indian greats through a flawed application of their so-called `proof by contradiction’, but they go on to make derogatory analyses as to why Indians could not `arrive’ at such mathematical arguments.

We are quoting such passages to exemplify the kind of narrow and limited thinking that is all around, and above which the advanced students of mathematics must raise themselves.

Robert Kaplan, in his popular book The Nothing That Is (OUP 2000), not only calls Brahmagupta’s rule \frac{0}{0} as “just out and out wrong” (p. 72) but also makes the amusing comments (pp. 73–74):

In their MacTutor article “A History of Zero”,⁷ J.J. O’Connor and E.F. Robertson (2000 CE) write in a more sober style:

Unfortunately, the above remarks get repeated in a subsequent article by R. Roy in a renowned journal of science education (Resonance, January 2003, p. 40):

In the context of statements like “The Indian mathematicians could not bring themselves to the point of admitting that one could not divide by zero.” or “Bhaskara still could not arrive at the modern concept that division by zero is not allowed.”, it is to be noted that ancient Indian mathematicians are indeed aware of the difficulties that zero can cause in the denominator and, therefore, do not allow division by zero in their treatises (or chapters) on arithmetic which are meant for the general public. It is usually in the chapters or books on algebra that rules on division by zero occur. And, as Avinash Sathaye remarks about algebra, it is “the first bold adventure into the unknown.”⁸

Nārāyaṇa (c. 1350 CE) clearly highlights the distinction made in the approach to division by zero between texts on arithmetic and texts on algebra. We quote from an English translation of his statement ([4], p. 239)

It may sound strange that a concept is treated as undefined in one branch and recognized in another. But are we not doing something similar? Do we not strictly forbid placing zero in the denominator at the level of basic school mathematics but uninhibitedly write 0^{-1}= \infty and \infty^{-1}=0 while pursuing an advanced, specialized and sophisticated topic in mathematics like valuation theory?

It is interesting to note that there is an awareness about the perils of zero in treatises on Indian philosophy—the concept is even compared with a snake which could be ruinous when wrongly grasped. In Mūlamadhyamakakārikā (Fundamental verses on the Middle Way), the foundational text of the Madhyamaka school of Mahāyāna Buddhist philosophy, the celebrated Buddhist philosopher Nāgārjuna (c. 150 CE) writes the following caveat (24.11; quoted in translation by C.K. Raju in [15], p. 170):

Division by Zero in Indian Mathematics: an Introduction

As pointed out earlier in this series (see [7], p. 57), ancient Indian mathematicians are usually very brief in their expositions. In particular, the full and explicit elaboration of their thinking on division by zero is not to be expected from their treatises, rather they have to be inferred from their examples and from the ancient commentaries on their works.

Modern historians try to interpret ancient Indian verses on division by zero in the light of calculus. Some of the statements then appear as imperfect, flawed expressions of calculus principles, and some examples appear as absurd errors. The picture changes in the light of algebra, as shown by Sathaye’s analysis of such (allegedly erroneous) examples from the algebra treatise of Bhāskarācārya. The algebraic interpretation is appropriate, as division by zero is usually discussed in the sections on algebra.

Some of the ancient Indian statements on division by zero in general mathematical texts (not the specialized algebra texts) can be interpreted in the framework of both algebra as well as calculus. The latter interpretation closely resembles the treatment of infinitesimals by Newton⁹ (not the formal modern analysis of Cauchy). Some statements have also been interpreted by C.K. Raju in the light of `non-standard analysis’ (see [15]). Our focus will be on the algebraic interpretations.

While Brahmagupta defines operations with zero and conceives of its reciprocal, Bhāskarācārya takes the bold step of not only defining zero-divided numbers but also admitting operations between such numbers and usual numbers. He is perhaps the first to do so.

We first recall the statements on zero-divided numbers by some of the prominent mathematicians preceding Bhāskarācārya. We shall then discuss Bhāskarācārya’s ideas separately.

Zero-Divided Numbers: From Brahmagupta to Śrīpati

The original verses of the Brāhma-Sphuṭa-Siddhānta (BSS in short) by Brahmagupta occur in [21]. As we highlighted in ([8]), BSS is the oldest extant treatise in the world (628 CE) which presents a comprehensive list of rules of operations with numbers – zero, negative and positive (Chapter 18 on algebra, v. 30–35). The topics listed in v. 2 of the chapter (i.e., topics under algebra) include khaṛṇadhana, i.e., (operations with) zero, negative and positive (numbers).

One of Brahmagupta’s statements (v. 34) is

After quoting excerpts from Brahmagupta’s khaṛṇadhana in his article on negative numbers, David Mumford writes ([12], p. 123):

The reader may note that Mumford calls Brahmagupta’s rule 0/0=0 an oddity, i.e., a strange or a peculiar rule (the only rule which does not match our modern rules), but not an error.

Unfortunately, the full text of Śrīdhara’s Pāṭīgaṇita (c. 750 CE) is not extant and his exposition on zero is lost (see [10], p. 151). However, Brahmagupta’s above statement is repeated in an ancient commentary on Śrīdhara’s Pāṭīgaṇita; the commentator explains the rule as (see [10], p. 150):

The Gaṇitatilaka (v. 45) by Śrīpati (1039 CE) too states that 0 \div 0=0 ([10], p. 150). Bhāskarācārya (1150 CE) does not seem to have made any statement on 0 \div 0.

For the division of a non-zero number by zero, Brahmagupta says (BSS XVIII, v 35):

Thus, Brahmagupta simply recommends leaving the fraction \frac{a}{0} as it is. He does not introduce operations \frac{a}{0}+\frac{b}{0} or \frac{a}{0}+b.

Similar statements occur in Chapter 14 (v. 6) of Śrīpati’s Siddhāntaśekhara (1039 CE), the chapter is titled Avyaktagaṇitādhyāya (chapter on algebra); the verse is quoted in ([10], pp. 153–154).

When a \ne 0, the fraction \frac{a}{0} is called taccheda or khacheda by Brahmagupta and khahara (zero-divided) by Śrīpati and Bhāskarācārya.

Khaguṇa and Khahara in Bhāskarācārya

We shall first highlight an algebraic structure formulated by Avinash Sathaye for understanding Bhāskarācārya’s treatment of zero and infinity, including the apparently erroneous examples in the algebra treatise Bījagaṇita, through his novel algebraic objects: khaguṇa (zero-multiplied) and khahara (zero-divided) numbers. An inspiration for Sathaye’s model comes from the multiple levels of infinities employed in modern commutative algebra and algebraic geometry. A crucial ingredient of this model is the concept of `idempotents’ in modern algebra. While historians of mathematics have tried to analyse Bhāskarācārya’s khahara in the framework of calculus, the difficulties with his examples in Bījagaṇita disappear in the algebraic interpretation based on idempotents.¹⁰

The concept of idempotents

An element e in a structure S equipped with a binary operation \circ is called idempotent if it satisfies e^2 =e, i.e., e\circ e=e.

In the ring of integers \mathbb Z, 0 and 1 are idempotents; in fact they are the only idempotents in \mathbb Z.

In the ring {\mathbb Z}/6{\mathbb Z} (comprising the six elements \overline{0}, \overline{1}, \overline{2},\overline{3}, \overline{4}, \overline{5}), the elements \overline{0}, \overline{1}, \overline{3} are the idempotents. (Since 9 \equiv 3 (modulo 6), {\overline{3}}^2=\overline{3} in this ring {\mathbb Z}/6{\mathbb Z}.)

The concept of idempotent is very useful in modern abstract algebra, especially ring theory. If a commutative ring R with unity contains an idempotent e apart from 0 and 1, then the ring can be decomposed into a product of two non-trivial rings.

The ring of n \times n matrices over any field, like the field of real numbers, contains several idempotents. Idempotent matrices arise frequently in statistical methods and applications, like regression analysis and linear models.

We shall see that in his treatise Bījagaṇita, Bhāskarācārya introduces numbers having the property of idempotence.

Khaguṇa and khahara

A khaguṇa is a new kind of number, different from usual real numbers, which is to be envisaged as a zero-multiplier but, in the spirit of postponement of evaluation, it is to be considered different from zero initially; it is only at the end that it can be equated to zero. The word khaguṇa literally means `zero-multiplied’ as well as `having qualities (properties) of zero’. A khahara is the reciprocal of a khaguṇa.

Since a subtle distinction would be made between the number zero and the khaguṇa, we introduce the notation \epsilon for the multiplier zero and \infty for the khahara 1/\epsilon, i.e., \epsilon \infty = 1= \infty \epsilon.

The verses of Bhāskarācārya lead to three kinds of numbers: {\mathbb R} \cup {\mathbb R}{\epsilon} \cup {\mathbb R}{\infty}:

1. usual real numbers x,
2. khaguṇa x \epsilon, where x \in {\mathbb R},
3. khahara x \infty, where x \in {\mathbb R}.

We have: (x \epsilon)(y \infty) =xy= (x \infty)(y\epsilon); \epsilon may be identified with zero at the end.

It is only in the commentary on Līlāvatī that Bhāskarācārya mentions applications in astronomy; there is no such comment in Bījagaṇita or in its commentary. His rules in the application-oriented general treatise Līlāvatī (v. 46; 47) can be interpreted from both viewpoints:

1. Algebra viewpoint: \epsilon is an algebraic object to be put to zero (only) at the end of all operations.
2. Calculus viewpoint: \epsilon is a variable infinitesimal t, t \rightarrow 0, resembling Newton’s fluxion.

His illustrative examples in Līlāvatī (v. 48) too agree well with both; but his exercises in Bījagaṇita (algebra) admit only the algebraic approach.

Though not explicitly mentioned by Bhāskarācārya, the examples in Bījagaṇita suggest that the khaguṇa \epsilon and khahara \infty are taken to be idempotents, i.e., {\epsilon}^2=\epsilon and {\infty}^2=\infty.

This interpretation was inferred independently by Sathaye and the present author. The idempotent property of zero (0^2=0) is mentioned just before the verse on khahara. Since the khaguṇa \epsilon represents (i.e., is to become) zero, it is natural to impart idempotence. Idempotence of \infty follows; besides it is natural to expect {\infty}^2=\infty.

the apparent difficulties with Bhāskarācārya’s examples in Bījagaṇita disappear in the algebraic interpretation based on idempotents.

The commentator Kṛṣṇa (c. 1600 CE) explicitly mentions the idempotent property of khahara. Sathaye and the present author were initially unaware of this statement in Kṛṣṇa’s commentary.

The idempotent property does not hold for the small numbers t in calculus tending to zero or the big numbers T tending to infinity. The property is used only in the examples of Bījagaṇita and not in Līlāvatī. In contrast with the examples in Līlāvatī, there is no suggestion of applications in Bījagaṇita.

We now quote a few verses from Bhāsakarācārya. The verses from Līlāvatī occur in [14] and [17]; the verses from Bījagaṇita occur in [1], [9] and [13].

Bhāskarācārya (1150 CE) defines the term khahara in Līlāvatī (v. 46, 47):

The same definition is given in Bījagaṇita (v. 2.18).

Thus, as long as the identification of khaguṇa \epsilon with zero remains `postponed’, one can recover x from x \epsilon by dividing the latter by the khaguṇa \epsilon.

However, once the khaguṇa gets equated to zero (i.e., once we arrive at x \times 0=0), x can no longer be recovered from that zero by dividing it by zero. This is strongly emphasised by Nārāyaṇa (c. 1350 CE) through the following simile (for the original verse, see [11], p. 11):

This statement amounts to saying that the principle of cancellation a \times c = b \times c \Rightarrow a=b is forbidden when c=0 (though it is allowed for c=\epsilon as long as \epsilon is not equated to zero).

We return to an earlier question: Suppose a \ne b are non-zero numbers. Are \frac{a}{0} and \frac{b}{0} to be treated as distinct or as the same?

Later commentators appear to differ. According to Gaṇeśa (c. 1548), \frac{a}{0} \ne \frac{b}{0}; but according to Kṛṣṇa (c. 1600), \frac{a}{0} = \frac{b}{0}.

No statement of equality is made by Brahmagupta or Bhāskarācārya. The interpretation (by default) would be that \frac{a}{0} \ne \frac{b}{0}.

However, in the model we have proposed, the two viewpoints may both be accepted through the principle of postponement of evaluation. They could be considered distinct at least initially and, at the end, they may be identified (if desired). For instance, in modular arithmetic, 1 and 8 are initially distinct (i.e., distinct integers) but get identified after going modulo 7.

Bhāskarācārya says (Bījagaṇita v. 2.20):

Thus, in our notation:
(I) x \infty +y =x \infty for all x,y \in {\mathbb R}.
(II) x \infty +y\epsilon =x \infty for all x,y \in {\mathbb R}.

The principle \infty +y=\infty can be seen in the extended real line of analysis, measure theory and the theory of rank one valuations in algebra.

The more general set {\mathbb R}\infty and the rule x \infty +y =x \infty can be seen in the study of higher rank valuations.

One may contrast this link of Bhāskarācārya’s rules to sophisticated branches of modern mathematics with the derisive remarks made around these rules by Kaplan et al.

We now summarize the formal algebraic structure propounded by Sathaye for placing Bhāskarācārya’s theory in a rigorous axiomatic mathematical framework.

The usual operations on \mathbb R are extended to the set {\mathbb R} \cup {\mathbb R}{\epsilon} \cup {\mathbb R}{\infty} such that for all x,y \in {\mathbb R},

1. x \infty +y =x \infty.
2. x \infty +y\epsilon =x \infty for all x,y \in {\mathbb R}.
3. (x \epsilon)(y \epsilon)=xy \epsilon.
4. (x \infty)(y \infty)=xy \infty.
5. (x \epsilon)(y \infty) =xy= (x \infty)(y\epsilon).

Note: Rule I or II is always to take precedence.

For instance,
(x\infty+y)^2=(x\infty)^2=x^2\infty,(x\infty+y)^2 \ne x^2{\infty}^2+2x{\infty}y+y^2=(x^2+2xy){\infty}.

Bhāskarācārya’s Examples

We first discuss two examples involving division by zero in Bhāskarācārya’s Bījagaṇita ([1], p. 35, [9], pp. 322–327). Neither example is included in the edition ([13]).

Example 1 in Bījagaṇita (v. 120)

In the usual reading by modern historians of mathematics: setting c=10000000, the equation becomes

\[\begin{align*}\{(\frac{x}{0}+c)^2+ (\frac{x}{0}+c)\}0=90\end{align*}\]or

\[\begin{equation}\label{Bijaganita1}
\frac{(x\infty+c)^2+(x\infty+c)}{\infty}=90
\end{equation}\]Interpreting \infty to be a variable T going to infinity, modern authors consider equation (\ref{Bijaganita1}) in the framework of conventional calculus as the statement
\underset{T \to \infty}{\lim} \frac{(xT+c)^2+(xT+c)}{T}=90.
The problem is then called absurd as LHS is infinite and RHS is not.

It is time to question whether the above approach is the only possible mathematical framework for Bhāskarācārya’s verse or the equation (\ref{Bijaganita1}). After all, the thinking of a master algebraist like him, who could solve difficult quadratic indeterminate problems like that of finding positive integers x,y satisfying 61x^2+1=y^2, must have been more nuanced.¹¹

We now consider the problem in the framework suggested by Sathaye. The steps, which are, in essence, the same as in Bhāskarācārya’s own commentary on the Bījagaṇita ([9], p. 322), are:

Let x denote the natural number being sought.

1. When zero-divided, it becomes x\infty.

2. When the quotient is augmented or reduced by 10000000, we get x\infty \pm 10000000.

3. Evidently, Bhāskarācārya’s clearly stated Rule I is meant to be applied here to obtain x\infty \pm 10000000 =x\infty .

4. On squaring, we get x\infty \cdot x\infty = x^2\infty by Rule IV.

5. Increasing by its own square root, one gets x^2\infty + x\infty, i.e., (x^2+x)\infty.

6. After the sum is zero-multiplied, we get (x^2+x)\infty \cdot \epsilon, i.e., (x^2+x), by Rule V.

7. Equating the above expression with 90, we obtain a familiar quadratic equation x^2+x=90.

8. As discussed in the previous part of this article (see [8], pp. 59–62), the formula for the roots of a general quadratic equation in one unknown, as well as the method of `elimination of the middle’ (or `completing the square’) to obtain the formula (i.e., the roots) is already expounded in the Indian treatises from at least the time of Brahmagupta. Applying them, the positive integral solution to the problem is x=9.

Note. If Rule I is not applied at the outset, one would get the equation x^2+2xc+x=90, with c=10000000, which is clearly not the intention.

We now discuss the second example on division by zero in Bhāskarācārya’s Bījagaṇita. Since the process has already been expounded in detail in the previous example, we now combine below, phrase by phrase, the verbal English translation with the corresponding (mathematical) symbolic formulation.

Example 2 in Bījagaṇita (v. 121)

Thus, the problem reduces to solving the quadratic equation 9x^2+12x=60 (with positive solution x=2).

In his commentary, Bhāskarācārya follows essentially the same steps (see [9], p. 325).

Note that, by conventional calculus, interpreting \epsilon to be a variable t going to zero, we would get a different equation, namely, \underset{t \to 0}{\lim} (\frac{9x^2}{4}t^2+3xt)\frac{1}{t}=15, i.e., 3x=15.

We now revisit an example from Bhāskarācārya’s Līlāvatī ([14], p. 49; [17], pp. 80–83) which can be interpreted in the formal algebra framework proposed by Sathaye, as well as through conventional calculus, both approaches yielding identical results.

Example in Līlāvatī (v. 48)

The first part asks a series of questions:

The answers are respectively:

1. 0+5=5.
2. 0^2=0, \sqrt{0}=0; 0^3=0, \sqrt[3]{0}=0
3. 5 \times \epsilon = 5 \epsilon =0; 10 \div 0 =\frac{10}{0}=10\infty.

The second part asks a problem to be formulated and solved. Again, we combine below the verbal English translation with the corresponding (mathematical) symbolic formulation:

Thus, the problem reduced to solving the simple linear equation (9/2)x = 63.

If however, we interpret khaguṇa in the spirit of calculus, i.e., as an infinitesimal tending to zero, then replacing \epsilon above by a variable t going to zero, we would have the formulation

\underset{t \to 0}{\lim}{ (\frac{9x}{2}\frac{t}{t}) =9x/2},which would yield the same equation (9/2)x = 63.

Ramanujan’s Thoughts on Zero and Infinity

P.C. Mahalanobis records certain thoughts on zero and infinity that Ramanujan shared with him, but which he did not understand. It appears that some of Ramanujan’s enigmatic statements may be envisaged as mathematical expressions which are symbolic of certain metaphysical principles.

Sathaye’s interpretation of Bhāskarācārya’s khahara appears to shed new light on the mathematical aspects of Ramanujan’s statements.¹²

For instance, the rule (x \epsilon)(y \infty) =xy= (y \infty)(x\epsilon) seems to be related to Ramanujan’s mysterious utterance that each finite number can be obtained as a particular product of infinity and zero.

Ramanujan (fourth from the left in the left photo) and Mahalanobis (fourth from the right in the right photo) met often during their overlap years in Cambridge. Their graduation photos reproduced here gives an idea of how they looked then.

Mahalanobis recalls ([16], pp. 82–83):

\blacksquare

References

• [1] S.K. Abhyankar (ed.), Bījagaṇita of Bhāskarācārya, Bhaskaracharya Pratishthana (1980).
• [2] S.S. Abhyankar, Historical Ramblings in Algebraic Geometry and Related Algebra, The American Mathematical Monthly, 83(6), 409–448 (1976).
• [3] N. Bourbaki, Elements of Mathematics: Commutative Algebra Ch. 1–7, Springer-Verlag (1989).
• [4] B. Datta and A.N. Singh, History of Hindu Mathematics Part I, Motilal Banarsidass (1935); reprinted by Asia Publishing House (1962) \& Bharatiya Kala Prakashan (2004).
• [5] A.K. Dutta, Mathematics in Ancient India Part 2: Computational Mathematics in Vedic and Sūtra Literature, Bhāvanā, 6(2), 43–61 (2022).
• [6] A.K. Dutta, Mathematics in Ancient India Part 3: The Decimal Notation and some Arithmetic Algorithms, Bhāvanā, 6(3), 50–67 (2022).
• [7] A.K. Dutta, Mathematics in Ancient India Part 5: The Kuṭṭaka Algorithm, Bhāvanā, 7(1), 52–67 (2023).
• [8] A.K. Dutta, Mathematics in Ancient India Part 6: The Foundations of Algebra – Glimpses, Bhāvanā, 7(2), 52–68 (2023).
• [9] Durgaprasad Dvivedi (ed.) Bijaganita of Bhaskaracharya, 2nd ed. Lucknow (1917).
• [10] R.C. Gupta, Zero in the Mathematical System of India, The Concept of Śūnya (eds. A.K. Bag and S.R. Sarma), Indira Gandhi National Centre for the Arts, Indian National Science Academy and Arya Book International, 148–158 (2003).
• [11] V.D. Heroor (ed.), Nārāyaṇa Paṇḍita’s Bījagaṇitāvataṁsa, Kavikulaguru Kalidas Sanskrit University (2019).
• [12] D. Mumford, What’s so Baffling About Negative Numbers? Studies in the History of Indian Mathematics (ed. C.S. Seshadri), Hindustan Book Agency, 113–143 (2010).
• [13] V.B. Panicker (ed.), Bhaskaracharya’s Bijaganitham, Bharatiya Vidya Bhavan (2006).
• [14] K.S. Patwardhan, S.A. Naimpally, S.L. Singh (eds.), Līlāvatī of Bhāskarācārya. Motilal Banarasidass (2001).
• [15] C.K. Raju, The Mathematical Epistemology of Śūnya, The Concept of Śūnya (eds. A.K. Bag and S.R. Sarma), Indira Gandhi National Centre for the Arts, Indian National Science Academy and Arya Book International, 170–183 (2003).
• [16] S.R. Ranganathan, Ramanujan: The Man and the Mathematician, Asia Publishing House (1967).
• [17] A.B. Padmanabha Rao, Bhāskarācārya’s Līlāvatī Part I, Chinmaya International Foundation Shodha Sansthan, Ernakulam (2015).
• [18] A.B. Padmanabha Rao, Ideas of Infinitesimals of Bhāskarācārya in Līlāvatī and Siddhāntaśiromaṇi, Indian Journal of History of Science 52(1), 17–27 (2017).
• [19] W. Rudin, Real and Complex Analysis, Tata McGraw Hill (1986).
• [20] A. Sathaye, Bhāskarācārya’s Treatment of the Concept of Infinity, Gaṇita Bhāratī 37(1-2), 55–67 (2015).
• [21] R.S. Sharma (ed.), Brāhma Sphuṭa Siddhānta of Brahmagupta. Indian Institute of Astronomical Research (1966).

Footnotes

1. Completion with respect to a metric is a technical mathematical notion used to ensure that `there are no points missing’ in the system under consideration. For example, placing only the rational numbers on the real number line will have the irrational numbers like \sqrt{2} or \pi on it missing; one `completes’ this to the real number line by including all the irrational numbers. The main tool here is the absolute value of a number, which gives its distance from the origin, and helps define a metric on the real line as the absolute value of the difference between any two numbers. ↩
2. In elementary textbooks on abstract algebra, the notation {\mathbb Z}_2 is sometimes used to denote the ring of integers modulo 2 (comprising just two elements), which is a different object altogether. ↩
3. For this article, Abhyankar was awarded the Chauvenet Prize of the Mathematical Association of America in 1978. It is awarded for an outstanding expository article on a mathematical topic. ↩
4. So widespread and deeply ingrained is the belief that it is absurd to consider division by zero that this perceived impossibility has led to a mathematical `Rajinikanth joke’. Rajinikanth is a popular Indian actor, and `Rajinikanth jokes’ are one-liners on impossible feats, like “Rajinikanth has counted to infinity, twice”, “Rajinikanth knows the last digit of \pi” and numerous such statements. One such one-liner is “Rajinikanth can divide by zero.”! During his lectures on the theme of this article, the present author has often suggested to Rajinikanth fans to remove the above item from the list as this feat does not need a Rajinikanth miracle, and that ancient Indian mathematicians like Brahmagupta and Bhāskarācārya have actually divided by zero!. ↩
5. strictly speaking, the images of elements of S in S^{-1}R. ↩
6. Details are given in [3], Chapter II, Section 2.1; in fact, in any basic text on commutative algebra. ↩
7. https://mathshistory.st-andrews.ac.uk/HistTopics/Zero/↩
8. See January 2022 issue of Bhāvanā, p.19. ↩
9. See [17], p. 80; [18]. ↩
10. After receiving an initial draft of the present article, M.D. Srinivas has pointed out to the author that Bhāskarācārya himself has worked out the examples in his commentary on the Bījagaṇita ([10], pp. 322–327) along essentially the same lines as what we have done in the next section, indicating that he implicitly followed the same principles that we had independently inferred and have formulated here. ↩
11. The intricacy of the problem can be seen from the fact that the smallest positive integer solution to the given equation is x=226153980, y=1766319049. This problem and a remarkable method for solving such problems is given by Bhāskarācārya in his Bījagaṇita (1150 CE), five hundred years before Fermat poses the problem as a challenge for contemporary mathematicians and to show them the beauty, the difficulty and the sophistication in the method of proofs in such problems on number theory. ↩
12. When Sathaye delivered a talk on the khahara at the Indian Statistical Institute, Kolkata, in 2014, Parthasarathi Mukhopadhyay of Ramakrishna Mission Residential College, Narendrapur (in West Bengal), had drawn our attention to these reminiscences on Ramanujan by Mahalanobis. ↩